Exercise : Solution of Questions on page Number :219
Answer 1:
If a ray of light goes from a rarer medium to a denser medium, it will bend towards the normal.
Answer 2:
If a ray of light goes form a denser medium to a rarer medium, it will bend away from the normal.
Answer 3:
When a beam of light travelling in a rectangular glass slab emerges into air, it bends away from the normal.

Answer 4:

When a beam of light travelling in air enters water, it bends towards the normal.
Answer 5:

When a ray of light travelling in water enters air, it bends away from the normal.
Answer 6:

When a ray of light travelling in air is incident on a parallel side glass slab, it bends towards the normal.
Answer 7:
We know that glass is a denser medium and air is a rarer medium. When a ray of light travels from a denser medium to a rarer medium, it bends away from the normal. So, the ray will bend away from the normal in the given case.
Answer 8:
We know that air is a rarer medium and water is a denser medium. When a ray of light goes from a rarer medium to a denser medium, it bends towards the normal. So, the light ray will bend towards the normal in the given case.
Answer 9:
When a ray of light travels from a denser medium (water) to a rarer medium (air), it bends away from the normal. Therefore, the ray of light will bend away from the normal in the given case.
Answer 10:
Refraction of light can cause following two effects:
1. An object placed under water appears to be raised.
2. A stick held obliquely and partly immersed in water appears to be bent at the water surface.
Answer 11:
This is due to the refraction of light.
Answer 12:
When a ray of light passes from air into glass, it bends towards the normal. So, the angle of refraction is smaller than the angle of incidence.
Exercise : Solution of Questions on page Number :220
Answer 13:
We know that air is a rarer medium and glass is a denser medium. When a ray of light goes from a rarer medium to a denser medium, it bends towards the normal. So, the light ray will bend towards the normal in the given case.
Answer 14:
Glass is denser than water; therefore, if light rays pass from water into glass, the rays will be refracted towards the normal.
Answer 15:
The speed of light in an optically rarer medium is more than the speed of light in a denser medium. Now, air is an optically rarer medium and glass is a denser medium. Therefore, light rays will travel fast in air.
Answer 16:
Refraction of light causes the water to appear shallower than it really is.
Answer 17:
True.
The refraction of light is due to the change in the speed of light on going from one medium to other. The speed of light ray slows down in denser materials.
Answer 18:
The bending of light, when it passes from one medium to another, is known as refraction. It occurs due to the change in the speed of light on going from one medium to another.
Answer 19:
(a) Light travelling along a normal is not refracted.
(b) Light bends when is passes from water into air. We say that it is refracted.
Answer 20:
Refraction of light:
The change in direction of light when it passes from one medium to another obliquely is called refraction of light.

Here, the light ray changes its direction or refracts at point A when it travels from air to glass. The ray changes its direction again at point B when it travels from glass to air.
Answer 21:

Here, light ray changes its direction or refracts at point A when it travels from air to glass. The ray changes its direction again at point B when it travels from glass to air.
The final direction of light ray, i.e., BX will be parallel to the direction OAY in which it enters the glass block.
Answer 22:
(a) Refraction of light ray when it passes from air to an optically denser medium:

(b) Refraction of light ray when it passes from an optically denser medium to air:

Answer 23:
(a)

NN’ is the normal at the point of entry.
(b) BC shows the path of light ray through the glass block and CD shows the path on the other side of the glass block.
Answer 24:
Angle of incidence:
The angle between the incident ray and the normal is called angle of incidence.
Angle of refraction:
The angle between the refracted ray and the normal is called angle of refraction.

Diagram for a refracted ray of light:
Here,
i = Angle of incidence
r = Angle of refraction
i’ = Angle of incident at the emergent point
r’ = Angle of refraction at the emergent point
Answer 25:
(a) Glass is optically denser as compared to water.
(Speed of light in an optically rarer medium is more than the speed of light in a denser medium.)
(b) If a ray of light passes from glass to water, it will bend away from the normal because glass is a denser medium as compared to water.
Answer 26:
(a) If the light ray hits the glass block at 90° (that is, perpendicular to the glass block):

(b) If it hits the glass block at an angle other than 90° (that is, obliquely to the glass block):

Answer 27:
When a ray of light travels from a rarer medium to a denser medium (air into glass), its speed decreases and it bends towards the normal.

Answer 28:”
(a) When a stick is half immersed in water, it appears to be bent at the surface due to the refraction of light.

In the above figure, the half portion BO of stick AO is immersed in water and it appears to be bent at point B. The light ray OC coming from the lower end O of the stick passes from water to air and gets refracted away from the normal in the direction CX. Another ray OD is refracted in the direction DY. These two refracted rays, i.e, CX and DY meet at point I, when produced backwards. The point I is nearer to the water surface than point O. Therefore, a virtual image of end O of the stick is formed as I.
So, human eye at position E sees the end O at I; i.e., stick appears to be bent.
(b) A coin in a glass tumbler appears to rise as the glass tumbler is slowly filled with water. It occurs because of refraction.
Answer 29:
(a) Refraction of light causes a tank full of water to appear less deep than it actually is.
Suppose, we have a tank of water as shown in the following figure.

Here, we take a point O at the bottom of the tank. We see this point because of the light rays coming from it. Now, a light ray OA from point O passes through water and enters air at point A. It gets refracted away from the normal in the direction AX. Similarly, another ray OB gets refracted at point B and bends away from the normal in the direction BY. These two refracted rays, i.e., AX and BY, meet at point I on producing them backwards. This point I (nearer to the water surface than O) is the image of point O.
Therefore, point O appears to be nearer at position I. Similarly, this can be applied to all points on the bottom of the tank. This causes the tank to appear less deep than it actually is.
(b) It is the phenomenon of refraction due to which a pencil partly immersed in water and held obliquely appears to be bent at the water surface.
Answer 30:
(a) In the following figure, a light ray travelling in air is incident on the rectangular glass slab. It gets refracted and bends towards the normal. Again, a change in the direction takes place when the refracted ray travelling in glass emerges into air. Here, the light ray bends away from the normal. We see that the incident and emergent rays are parallel to each other. These rays are parallel because the extent of bending on the opposite and parallel faces of slab is equal and opposite.

(b) The perpendicular distance between the original path of the incident and emergent rays coming out of the glass slab is called lateral displacement of the emergent ray. It is shown in the above figure.
(c) Two factors on which the lateral displacement of the emergent ray depends:
1. Angle of incidence
2. Thickness of glass slab
Answer 31:

In the above figure, the portion BO of the pencil AO is immersed in water and it appears to be bent at point B. The light ray OC coming from the lower end O of the pencil passes from water to air and gets refracted away from the normal in the direction CX. Another ray OD is refracted in the direction DY. These two refracted rays, CX and DY, meet at point I, when they are produced backwards. Point I is nearer to the water surface than point O. Therefore, a virtual image of end O of the pencil is formed as point I.
So, human eye at position E sees the end O at point I; i.e., pencil appears to be bent.
If water is replaced by another liquid, which is optically denser than water, the bending of pencil will increase because an optically denser medium causes more refraction or more bending of light rays.
Exercise : Solution of Questions on page Number :221
Answer 32:
(d) is not refracted
When a ray of light travels along the normal incident on the surface, it is not refracted.
Answer 33:
(b) greater than the angle of incidence
Because when a light ray passes from denser medium to a rarer medium, it bends away from the normal.
Answer 34:
(b) smaller than the angle of incidence
Because when a light ray passes from a rarer medium to a denser medium, it bends towards the normal.
Answer 35:
The speed of light in air is:
(d) 3 × 108 m/s
Answer 36:
(d) it is refracted away from the normal
Because if a ray of light goes form a denser medium (glass) to a rarer medium (water), it bends away from the normal.
Answer 37:
(c) does not get refracted
When a ray of light travels along the normal incident on the boundary separating two mediums, it is not refracted.
Answer 38:
(c) 90°
If a ray of light travels along the normal, it is not refracted.
Answer 39:
Diagram E shows that the ray of light is refracted correctly.
When a light ray goes from a rarer medium to a denser medium, it bends towards the normal. This principle is replicated in this diagram.
Answer 40:
(a) Angle of incidence is 00 because light ray is travelling along the normal and angle of incidence is the angle between the light ray and the normal.
(b) If the incident ray falls normally on the surface, no bending of light ray takes place. So, the angle of refraction is also 00.
Answer 41:
In case of reflection, the angle of reflection is equal to the angle of incidence. On the other hand, in case of refraction, the angle of refraction is not equal to the angle of incidence.
Answer 42:
(a) To produce a large amount of bending, the light ray has to enter the glass with a large angle of incidence.
(b) For no refraction, the light ray has to enter the glass perpendicularly.
Answer 43:
(a) We can bend light away from the normal by making the light to enter from a denser medium to a rarer medium.
(b) Light will not refract if it travels at the right angles to the surface of the substance.
Answer 44:

When a beam of light rays enters the glass block, it gets refracted. It bends towards the normal. Also, when these light rays leave the block, they bend away from the normal.

When light rays fall normally on the surface of the glass block, there is no bending of rays; the rays travel straight.
Answer 45:
When a beam of light enters glass at an angle, the speed changes and therefore the direction of light changes; i.e., bending of light occurs.
When a beam of light falls at right angles to the surface of glass, all parts of light waves reach the glass at the same time, enter the glass at the same time and hence slow down at the same time. Due to this no change in direction of light takes place; i.e., bending of light does not take place.
Exercise : Solution of Questions on page Number :227
Answer 1:
The ratio of sine of angle of incidence to the sine of angle of refraction is a constant value. This value is called the refractive index of a medium.
Answer 2:
The relationship between the angles of incidence and refraction is given by Snell’s law.
According to this law, the ratio of the sines of the angles of incidence and refraction is constant for a given pair of media.
Sine of angle of incidence (i)Sine of angle of refraction (r)=constant (n )This constant is known as refractive index.
Exercise : Solution of Questions on page Number :228
Answer 3:
Refractive index is a ratio of two similar quantities (the sines of angles); therefore, it has no units.
Answer 4:
The refraction index of glass is higher than that of water. We know that a denser medium has high refractive index. Glass is denser than water; therefore, it has high refraction index.
Answer 5:
Carbon disulphide is optically denser than ethyl alcohol. It is because a substance with high refractive index is optically denser than the other with low refractive index.
Answer 6:
The refractive index of diamond is 2.42. This means that the ratio of the speed of light in air (or vacuum) to that in diamond is equal to 2.42.
Answer 7:
Here,
ndiamondair =2.42nair diamond =1 airndiamond=12.42=0.41
Answer 8:
The refractive index is the ratio of speeds of light in the two mediums.
The refractive index of medium 2 with respect to medium 1 is equal to the ratio of speeds of light in medium 1 and in medium 2.
n12=v1v2v1=Speed of light in medium 1v2=Speed of light in medium 2
Answer 9:
When a ray of light goes from air into a clear material, you see the ray bend. How much the ray bends is determined by the refractive index of the material.
Answer 10:
Three examples of materials that refract light are glass, water and air.
The speed of light rays changes when they enter these materials.
Answer 11:
According to Snell’s law, the ratio of sines of the angles of incidence and refraction is constant for a given pair of mediums.
We get:
sin i /sin r = n (constant)
This constant is called refractive index.
According to the question:
Angle of incidence, i = 60°
Angle of refraction, r = 32.7°
Refractive index, n = ?
Applying the above formula, we get:
sin i / sin r = n
or, n = sin 60°/ sin 32.7°
= 0.866/0.540 = 1.60
Thus, the refractive index of glass is 1.60.
Answer 12:
(a) Absolute refractive index of flint glass = Speed of light in vacuum / Speed of light in flint glass
= (3.00 × 108) / (1.86 × 108)
= 1.61
Absolute refractive index of crown glass = Speed of light in vacuum / Speed of light in crown glass
= (3.00 × 108) / (1.97 × 108)
= 1.52
(b) Relative refractive index for light going from crown glass to flint glass is given by:
(Speed of light in crown glass) / (Speed of light in flint glass)
= (1.97 × 108) / (1.86 × 108)
= 1.059
Answer 13:
(a) air nx = (Speed of light in air) / (Speed of light in medium X)
= (3 × 108) / (2 × 108)
= 1.5
(b) air n Y = (Speed of light in air) / (Speed of light in medium Y)
= (3 × 108) / (2.5 × 108)
= 1.2
(c) x nY = (Speed of light in medium X) / (Speed of light in medium Y)
= (2 × 108) / (2.5 × 108)
= 0.8
Answer 14:
Given:
Speed of light in air = 3,00,000 km/s
Refractive index of the medium = 6/5
Speed of light in the given medium = ?
Applying formula for refractive index, we get:
Refractive index of a medium = (Speed of light in air) / (Speed of light in medium)
or
(Speed of light in medium) = (Speed of light in air) / (Refractive index of a medium)
= 3,00,000 / (6/5)
= 2,50,000 km/s
Answer 15:
Given:
Refractive index of glass = 1.5
Speed of light in air = 3.0 × 108 m/s
Speed of light in glass = ?
Applying the formula for refractive index, we get:
Refractive index of a medium = (Speed of light in air)/(Speed of light in medium)
For glass:
Refractive index of glass = (Speed of light in air)/(Speed of light in glass)
(Speed of light in glass) = (Speed of light in air)/(Refractive index of glass)
= (3.0 × 108)/1.5
= 2.0 × 108 m/s
Answer 16:
Given:
Speed of light in water = 2.25 × 108 m/s
Speed of light in vacuum = 3 × 108 m/s
Refractive index of water = ?
Applying the formula for refractive index, we get:
Refractive index of a medium = (Speed of light in vacuum) / (Speed of light in the medium)
For water:
Refractive index of water = (Speed of light in vacuum) / (Speed of light in water)
= (3 × 108) / (2.25 × 108)
= 1.33
Thus, the refractive index of water is 1.33.
Answer 17:
We know:
Refractive index = Speed of light in air/Speed of light in diamond
Let X be the speed of light in diamond. Now, we have:
2.42 = 3.0 × 108 ms-1 / X
X = 1.24 × 108 ms-1
Answer 18:
(a)
Laws of refraction of light:
(i) The incident ray, the refracted ray and the normal to the interface of two transparent mediums at the point of incidence, all lie in the same plane.
(ii) The ratio of sines of angles of incidence and refraction is a constant, for the light of a given colour and for a given pair of mediums. This law is also known as Snell’s law of refraction.

(b)
If i is the angle of incidence and r is the angle of refraction, we get:
sin isin r = constant
This constant value is called the refractive index of the medium in which light enters through air. Refractive index of a medium can also be expressed in terms of speed of light as follows:
1n2 = speed of light in medium 1speed of light in medium 2
Here, 1n2 is the refractive index of medium 2 with respect to medium 1.
(c)
Here, mediums 1 and 2 are air and water, respectively.
Now,
Refractive index of water = Speed of light in air / Speed of light in water
= 300 million ms−1 / 225 million ms−1
= 1.33
Therefore, refractive index of water is 1.33.
Answer 19:
(d) S
Explanation:
We know that:
Refractive index (n) = Speed of light in air / Speed of light in a medium
According to this formula, speed of light will be maximum in a substance whose refractive index is minimum.
Therefore, speed of light will be maximum in substance S whose refractive index is 1.31.
Answer 20:
(c) material C
Explanation:
The refraction in a material depends on its refractive index. Refractive index is calculated by sin isin r.
This ratio is maximum for material C; therefore, it produces maximum refraction.
Exercise : Solution of Questions on page Number :229
Answer 21:
(c) 4/6
Explanation:
Refractive index of material 2 with respect to material 1 is given by:
1n2 = Speed of light in medium 1Speed of light in medium 2
By the same argument, refractive index of medium 1 with respect to medium 2 is given by:u
2n1 = Speed of light in medium 2Speed of light in medium 1 = 1/1n2
1n2 is 32; therefore, 2n1 will be 1/32.
Therefore, refractive index of light going from glass to air will be 4/6.
Answer 22:
(c) in medium C
Refractive index = sin isin r
The angle of incidence is equal in all the cases; therefore, the refractive index will be maximum in the case of minimum angle of refraction. According to this argument, medium C will have minimum angle of refraction because it has maximum refractive index.
Answer 23:
(a) 2.4
Refractive index = Speed of light in airSpeed of light in the substance
=3×108 m/s1.25 ×108 m/s = 2.4
Answer 24:
(d) substance S
Refractive index = sin isin r
The value of (Sin i) is same in all the cases; therefore, the value of (Sin r) will be maximum for minimum refractive index. This means that the angle of refraction will be maximum for minimum refractive index and substance S has minimum refractive index.
Answer 25:
(a) 1.33
Explanation:
Velocity of light in water = 225,563,010 m/s
Velocity of light in air = 300,000,000 m/s
Refractive index = Velocity of light in air Velocity of light in water
= 300,000,000225,563,010=1.33.
Answer 26:
(c) 0.75
Explanation:
Refractive index of air with respect to water = 1Refractive index of water with respect to air
Refractive index of air with respect to water = 3/4 = 0.75
Answer 27:
(d) carbon disulphide
Explanation:
Refractive index = Speed of light in airSpeed of light in medium
Speed of light in the medium is slowest; therefore refractive index will be maximum as the speed of light in air is constant. Thus, light will travel slowest in the substance with refractive index 1.63.
Answer 28:
(d) 1.125
Explanation:
Refractive index of glass with respect to water = Refractive index of glass with respect to airRefractive index of water with respect to air
= 3243
=1.125
Answer 29:
(1) Light speeds up as it travels from a denser medium to a rarer one, ie., from a medium with a higher refractive index to one with a lower refractive index. An example would be the medium pair of diamond and water, where light travels from diamond to water.
(2) Using the same argument, light slows down as it travels from a medium with a lower refractive index to one with a higher refractive index. Therefore, the medium pair of crown glass and ruby can be taken as an example, where light moves from the crown glass to the ruby.
Answer 30:
The refractive index of a medium is related to the speed of light as follows:
Refractive index =speed of light in vacuum speed of light in a medium
Since the speed of light in vacuum is a constant, the refractive index becomes inversely proportional to the speed of light in a medium.
(1)The speed of light is maximum in a medium that has the lowest refractive index, ie., medium A .
(2) The speed of light is minimum in a medium that has the highest refractive index, ie., medium D.
Exercise : Solution of Questions on page Number :239
Answer 1:
A convex lens can concentrate the sun’s rays to a point and burn a hole in a piece of paper.
Answer 2:
The usual name for a point inside a lens through which light passes undeviated is the optical center .
Answer 3:
The height of the image formed is 1 cm. The reason being, when an object is placed at a distance of 2f from a convex lens, the size of the image formed is equal to the size of the object .
Answer 4:
The image will be formed at a distance of 2F from the lens and behind it.
Answer 5:
For an object placed at the focus of a convex lens, the image is formed at infinity.
Answer 6:
The object should be placed at a distance that is less than F(focus) of the lens. The resultant image is virtual, erect and enlarged and formed on the same side as the object. From this theory, a convex lens can therefore, be used as a magnifying glass.
Answer 7:
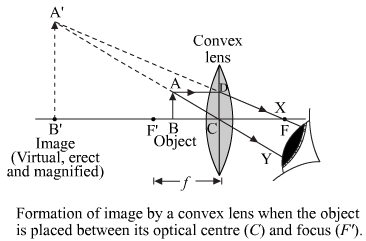
The object should be placed between the optical centre and the focus of a convex lens to obtain a virtual, erect and magnified image.
Answer 8:
The object should be placed between f and 2f of a convex lens to obtain a real, inverted and magnified image.
Exercise : Solution of Questions on page Number :240
Answer 9:
When an object is placed beyond 2F, the image formed is real and diminished.
Answer 10:
The image will be formed at the focus.
Answer 11:
The ray will pass through the focus after refraction since it is parallel to the principle axis. See the diagram given below.

Answer 12:
For a magnifying glass, we use a convex lens. The object should be placed between the optical centre and the focus.
Answer 13:
Focal length depends on :
(1) Curvature of the lens
(2) Material medium of the lens
Answer 14:
Two uses of convex lenses are:
(1) They are used in telescopes.
(2) The convex lenses are used in magnifying glasses.They are used in the glasses of those affected with long-sightedness.
Answer 15:
(a) Parallel rays of light are refracted by a convex lens to a point called the focus.
(b) The image in a convex lens depends upon the distance of the object from the lens.
Answer 16:
A lens is an optical device that transmits and refracts a ray of light, and converges or diverges a beam of light.
| Convex Lens | Concave Lens |
| 1.A convex lens converges or concentrates the light rays to a point. 2.A convex lens has a real focus. 3.A convex lens has a positive focal length. |
1. A concave lens diverges the rays passing through it. 2.A concave lens has a virtual focus. 3.A concave lens has a negative focal length. |
A convex lens is a converging lens .
Answer 17:
A convex lense is outwardly curved and causes the light to pass through it and converge or concentrate to a point. Think of a magnifying glass that is used to burn something. The light that passes through it concentrates to a point, and this convergence is used to burn things. See the diagram given below.

(b) An imaginary line that passes through the optical center and the center of curvature of both faces of the lens, and is perpendicular to the faces is known as the principal axis.
Focus is a point on the principal axis where all the rays that are parallel to the principal axis meet after refraction from the lens.
The focal length may be defined as the distance between the optical centre and principal focus of the lens.
Answer 18:
(a) A concave lense is curved inwards. Light passing through it diverges or spreads out. Think of a flashlight. The light from the small bulb passes through the lens and spreads out into a bigger beam on the other side. See the diagram given below.

(b) The principle focus of a concave lens is a point on the principle axis where all the reflected rays parallel to the principle axis appear to diverge.
Answer 19:

A convex lens forms a real and magnified image when an object is placed between F and 2F.
Answer 20:
The diagram shows the formation of the image of a finite object that is placed in front of a convex lens and between f and 2f:

Two characteristics of the image so formed are:
(1) It is Real
(2) It is Inverted
Answer 21:
Image formation diagram:

Characteristics of the image so formed:
1) On the same side as that of the object or behind the lens
2) Nature of image – virtual and erect
3) Size of Image – enlarged
Answer 22:
Image formation diagram:

Two characteristics of the image formed:
(1)Nature of image – real and inverted.
(2)Size of Image – same size as that of object .
Answer 23:
Image formation diagram:

Image properties:
Image – between F and 2F
Nature of image – real and inverted
Size of Image – diminished
Answer 24:
Image formation diagram:

Image characteristics:
(1)Image – at F
(2)Nature of image – real and inverted
(3)Size of Image – point sized
Answer 25:
(a) It’s a convex lens. The light rays will converge at a point called the focus of the lens.

(b) A convex lens converges a beam of light rays at a point called the focus. Therefore, it is unwise to look at the sun as it may damage the eye.
Answer 26:
For a convex lens:
(a) To form a real, inverted and smaller image than the object, the object should be beyond 2F.
(b) To form a real and inverted image, which is the same size as that of the object, the object should be at 2F.
(c) To form a real, inverted and larger image than the object, the object should be between F and 2F.
(d) To form a virtual, upright and larger image than the object, the object should be between F(focus) and the optical centre.
Answer 27:
Magnifying glass:

A lens with a short focal length is used so as to obtain greater magnification.
Answer 28:
To determine the focal length of a convex lens, place the convex lens in a holder and keep it in front of a distant object like a tree. A cardboard screen is put behind the lens. Now, change the distance of the screen from the convex lens until a clear, inverted image of the tree is formed on the screen. Measure the distance of the screen from the lens. This distance will be the focal length of the convex lens. Here, we have used the fact that the image of an object at a far distance is formed at the focus of a convex lens.
Answer 29:
(a) Suppose that a parallel beam of light rays falls on a convex lens as shown in the figure. These light rays are parallel to one another and also to the axis of the lens. The incident rays pass through the convex lens and get refracted according to the laws of refraction. All the rays, after passing through the convex lens, converge at the same point F, on the other side of the lens. The point F is called the principal focus of the convex lens. Thus, the point of convergence of the parallel beam of light rays to a single point is called the focus of the lens.

(b) A convex lens has a real focus because all the light rays actually pass through the focus.
(c) Some things that a convex lens and a concave lens have in common are as follows:
Both converge a parallel beam of light rays to a single point called the focus.
Both form real and inverted images of an object, except in one case.
Both form a virtual, erect and magnified image when the object is placed between the pole and focus of a convex mirror, and between the centre of the lens and the focus, for a concave lens./images/Lakhmir-physics/
Exercise : Solution of Questions on page Number :241
Answer 30:
(a) When a parallel beam of light rays falls on a concave lens, the rays spread out (or diverge) after passing through the lens (figure). Since the refracted rays are diverging away from one another, they do not actually meet at a point. The diverging rays, when produced backwards appear to meet at a point F called the principal focus, on the left side of the lens.

(b) A concave lens has a virtual focus because the light rays do not actually pass through the focus.
(c) Few things which are common to both a concave lens and a convex mirror are as follows:
Both diverge a parallel beam of light.
Both form a virtual image at all times.
Both have a virtual focus.
Answer 31:
(a) When an object is placed at 2F1 , the image so formed by the convex lens is at 2F. This image is real, inverted and of same size as the object as shown in the figure.

(b) When the object is placed between F1 and the optical centre O of the lens, the image formed by the convex lens is behind the object (left of the lens). This image is virtual, magnified and erect as shown in the figure.
Case (b) is used as a magnifying glass. The reason being, the image of an object that is placed between F1 and the optical centre is virtual and magnified, which is the requirement of a magnifying glass.
Answer 32:
(a) When an object is placed well outside the principal focus of a convex lens, the image formed is real and inverted as shown in the figure.

(b)
(i) When an object is moved towards the lens, the size of the image starts increasing. The position of the image starts moving away from the lens and towards infinity (on the right side of the lens) till the object is placed at the focus. When the object is moved closer to the lens, the image is formed behind the object (left side of the lens). This image is virtual, erect and magnified in size.
(ii) When the object is moved away from the lens, the image so formed moves closer to the lens and gets diminished in size.
Answer 33:
(a) A virtual image is an image formed by a lens/mirror that cannot be taken on a screen. A magnified image implies that the size of the image formed is larger than the size of the object.
(b) When an object is placed between the focus and the optical centre of a convex lens, the image formed is virtual and magnified as shown in the figure.

(c) We will choose the lens with a focal length of 4 cm as the image formed will be more magnified (the smaller the focal length of a lens, the higher will be the magnification).
Answer 34:
(a) A real image can be projected on a screen unlike a virtual image because a real image is formed by the actual meeting of light rays. A virtual image, on the other hand, is not formed by the actual meeting of light rays.
(b) When an object placed is beyond 2F’, the image formed by the convex lens is real and diminished, as shown in the figure.

(c) A camera works on the above arrangement because the camera lens produces a small, real and inverted image of an object on the film.
Answer 35:
(b) 7 cm,
since the image of an object placed between the focus and the optical centre of a convex lens is enlarged and virtual.
Answer 36:
(d) Clay,
since it is opaque and does not let light to pass through it.
Answer 37:
(c) A parallel beam of light
The reason being, a beam of light coming from the focus of a converging lens becomes parallel, after refraction from the lens.
Answer 38:
(b) Inverted and magnified
For an object placed between the focal length and twice the focal length of a converging lens, the image formed is real, inverted and magnified.
Answer 39:
(a) Real, larger than the object
The reason being, the image of an object placed between f and 2f of a convex lens is real, inverted and magnified.
Answer 40:
(c) Concave mirror as well as Convex lens
When an object is placed at the focus of a concave mirror (convex lens), the reflected (refracted) light rays are always parallel to each other and to the principal axis.
Answer 41:
(c) More than 15 cm but less than 30 cm
For an object placed between F and 2F of a convex lens, the image formed is real and enlarged.
Answer 42:
(c) Increase the distance of the object from the lens
As the object moves away from the lens, the image gets closer to the lens.
Answer 43:
(d) 32 cm
A convex lens forms a real image at 2f on the right side of the lens. The size of the image is equal to that of the object if the object is placed at 2f.
∴ Distance between the image and the object = 2f + 2f = 4f = 4 × 8 = 32 cm
Answer 44:
(b) between F and optical centre
When an object is placed between F and the optical centre, the image of the object formed by convex lens is virtual, erect and magnified.
Answer 45:
(c) at 2f
This is because a convex lens produces an image of the same size as the object when the object is placed at 2f.
Answer 46:
(b) 16 cm
Given:
Magnification, m = 3
Focal length f = 12 cm
Image distance v = ?
Object distance u = ?
We know that:
m = vu
Therefore
3 = vu
3u = v
Putting these values in lens formula, we get:
1v-1u=1f⇒ 13u-1u=112⇒ 1-33u=112⇒ -23u=112⇒ 3u=-24
⇒ u=-243⇒ u=-6 cm
v = 3u = -8 × 3 = -24 cm
Here, minus sign show that image is formed on the left side of the lens.
Distance between image and object = 24 – 8 = 16 cm.
Answer 47:
(b) 10 cm
We know that a converging lens forms an image of same size as object when object is placed at a distance of 2f from the lens. It is given that the image is smaller than the object if object is kept at a distance of 21 cm. Similarly, the image is bigger than the object if object is kept at a distance of 19 cm. Therefore, at 20 cm, the distance should be 2f. This means that the focal length is approximately 10 cm.
Answer 48:
(a) The object should be placed at 20 cm because a convex mirror forms a real, magnified image when an object is placed between f and 2f.
(b) The object should be placed at 10 cm because a convex mirror forms a virtual, magnified image when an object is placed placed between f (focus) and the optic centre.
(c) The object should be placed at 35 cm because a convex mirror forms a real, diminished image when an object is placed beyond 2f.
(d) The object should be placed at 30 cm because a convex mirror forms a real image of the same size when an object is placed at 2f.
Answer 49:
(a) The image will be virtual and magnified. We know that a convex lens forms the image of the same size as that of the object when the object is placed at 2f. Thus, the focal length of the lens is 13 cm. In this case, the object is placed between the focus (f) and the optic centre. This position of the object results in the formation of a virtual and magnified image.
(b) The image will be real and magnified because the object is placed between 2f and f.
Answer 50:
(a) When rays of light from a distant object pass through a converging lens, the light rays converge at the focus of lens (fig.).

(b) When rays of light come from the focus of lens, the emergent rays of light get parallel to the principal axis.
Exercise : Solution of Questions on page Number :246
Answer 1:
Lens formula is:
1/v-1/u=1/f
Now, the mirror formula is given by:
1/v+1/u=1/f
In both the formulas,
u = Object distance
v = Image distance
f = Focal length
In mirror formula, positive sign is present between the reciprocals of image distance and object distance .
In lens formula, negative sign is present between the reciprocals of image distance and object distance.
Answer 1:
Lens formula is:
1/v-1/u=1/f
Now, the mirror formula is given by:
1/v+1/u=1/f
In both the formulas,
u = Object distance
v = Image distance
f = Focal length
In mirror formula, positive sign is present between the reciprocals of image distance and object distance .
In lens formula, negative sign is present between the reciprocals of image distance and object distance.
Answer 3:
If the magnification produced by the lens is +3, the image will be virtual and erect. It is because if the magnification has a positive value, the image is always virtual and erect.
Answer 4:
If the magnification produced by the lens is -0.5, the image will be real and inverted. It is because if the magnification produced by a lens has a negative value, the image is always real and inverted.
Answer 5:
Given:
Object distance, u = -10 cm
(Since object is always placed on the left side of lens)
Focal length, f = +10 cm
According to lens formula:
1/v-1/u=1/f
1/v=1/f+1/u
1/v=1/10+ (-1/10)=0
v=1/0=∞
Therefore, the position of image will be at infinity.
Answer 6:
Given:
u = -30 cm
(Since the object is placed on the left side of lens)
Focal length f = +15 cm
According to lens formula:
1/v-1/u=1/f
or 1/v=1/f+1/u=1/15+(-1/30 )or v=30 cm
Thus, the image is formed at a distance of 30 cm from the convex lens. The plus sign for image distance shows that the image is formed on the right side of the convex lens. Only real and inverted image is formed on the right side of a convex lens; therefore, the image is real and inverted.
Answer 7:
Magnification 1 means that the image distance is the same as the object distance and the size of image is the same as the object.
This happens only if the position of the object is at 2f, i.e, 2 × focal length.
Thus, the object must be placed at a distance of 24 cm (2 × 12) from the converging lens.
Answer 8:
New Cartesian Sign Convention for spherical lenses:
New Cartesian Sign Convention is used for measuring various distances in the ray diagrams of lenses.
According to this convention:
I. Object is always placed on the left of the lens; i.e., light must fall on the lens from left to right.
II. All distances parallel to the principal axis are measured from the optical centre of the lens.
III. The distances along the direction of incident rays (along positive x-axis) are taken as positive, whereas the distances opposite to the direction of incident rays (along negative x-axis) are taken as negative.
IV. Distances measured above the principal axis (along positive y-axis) are taken as positive.
V. Distances measured below the principal axis (along negative y-axis) are taken as negative.
Answer 9:
Given:
Object distance, u = -10 cm (It is to the left of the lens.)
Focal length, f = + 20 cm (It is a convex lens.)
Putting these values in the lens formula, we get:
1/v – 1/u = 1/f (v = Image distance)
1/v – 1/(-10) = 1/20
or, v = -20 cm
Thus, the image is formed at a distance of 20 cm from the convex lens (on its left side).
Only a virtual and erect image is formed on the left side of a convex lens. So, the image formed is virtual and erect.
Now,
Magnification, m = v/u
m = -20 / (-10) = 2
Because the value of magnification is more than 1, the image will be larger than the object.
The positive sign for magnification suggests that the image is formed above principal axis.
Height of the object, h = +4 cm
magnification m=h’/h (h=height of object)
Putting these values in the above formula, we get:
2 = h’/4 (h’ = Height of the image)
h’ = 8 cm
Thus, the height or size of the image is 8 cm.
Answer 10:
Given:
Focal length, f = 5 cm
Image distance, v = -25 cm (Image is virtual.)
Applying the lens formula, we get:
1/v – 1/u = 1/f (u = Object distance)
1/(-25) – 1/u = 1/5
or, 1/u =1/(-25)-1/5
or, 1/u =-6/25
or,
u = -25/6 cm
Now,
Magnification, m = v/u
= (-25)/(-25/6)
= +6
Answer 11:
Given:
Height of the object, h = 5 cm
Object distance, u = -10 cm
Focal length, f = 6 cm
Applying lens formula, we get:
1/v – 1/u = 1/f (v = Image distance)
1/v – 1/(-10) = 1/6
or, v = 15 cm
Thus, the image is formed at a distance of 15 cm behind the convex lens (on the right side).
Now
Magnification, m = v/u = h’/h
m = 15/(-10)
m = -1.5
The value of magnification is negative; therefore, the image will be real and inverted.
Answer 12:
Given:
Object distance, u = -20 cm
Image distance, v = -50 cm (Image is virtual.)
Applying lens formula, we get:
1/v – 1/u = 1/f (f = focal distance)
1/(-50) – 1/ (-20) =1/f
or, 1/f = (-2+5)/100
or, 1/f =3/100
or, f = 100/3 = + 33.3 cm
Thus, the focal length of convex lens is 33.3 cm.
Answer 13:
Given:
Object distance, u = -100 cm
Focal length, f = 40 cm
Applying lens formula, we get:
1/v -1/u = 1/f
1/v – 1/(-100) = 1/40
or, 1/v = 1/40 – 1/100
or, 1/v = 6/400
or, 1/v = 3/200
or, v = 200/3 = +66.6 cm
Now
Magnification, m = v/u
m= (200/3)/(-100)
m= -2/3
(i) The value of magnification is negative, therefore, the image will be real and inverted.
(ii) The value of v is (+66.6 cm); therefore, the image is formed 66.6 cm behind the convex lens.
Answer 14:
Given:
Object distance, u = -15 cm
Magnification, m = -3 (Image is inverted.)
Applying magnification formula, we get:
m = v/u = -3
or, v/(-15) = -3
or, v = 45 cm
Applying lens formula, we get:
1/v – 1/u = 1/f
1/45 – 1/(-15) = 1/f
1/f = ( 1+3 )/45
1/f = 4/45
or f = 11.2 cm
Hence, focal length of the lens is 11.2 cm.
Exercise : Solution of Questions on page Number :247
Answer 16:
Given:
Object distance, u = -25 cm
Focal length, f = 10 cm
Height of the object, h = 5 cm
Applying the lens formula, we get:
1f=1v-1u
⇒1v=1f+1u⇒1v=1f+1u⇒ 1v=110+1-25⇒ 1v=5-250⇒ 1v=350⇒ v=503=+16.6 cmThe image will be at a distance of 16.6 cm behind the lens. m=vu=503m=h,h=h,5h,5=-23 h,=-103=-3.3 cmThus, the image will be 3.3 cm high. It will be real and inverted.

Answer 17:
Given:
Focal length, f = 18 cm
Image distance,v = 24 cm
Putting these values in lens formula, we get:
1/v – 1/u = 1/f
or, 1/u = 1/v -1/f
or, 1/u = 1/24 -1/18 = -(1/72)
or, u = -72 cm
Thus, the object should be placed at a distance of 72 cm from the lens.
Now
Magnification, m = v /u = 24/ (-72) = -(1/3)
Answer 18:
Given:
Size of object, h = 2 cm
Focal length, f = 5 cm
Object distance, u = -10 m = -1000 cm
Applying lens formula, we get:
1/v – 1/u = 1/f
1/v = 1/f + 1/u
or, 1/v = 1/5 + 1/(-1000)
or, 1/v=(-200+1)/-1000
or, 1/v=199/1000
or, 1/v=1000/199
or, v=5 cm (approx)
Image distance, v =5 cm behind the lens;
Applying magnification formula, we get:
m = h’/h = v/u
m = h’/2 = (5)/(-1000)
or, h’ = -0.01 cm
Thus, the size of the image is 0.01 cm.
So, the image is inverted (h’ is negative), highly diminished (h’ is smaller than h) and real (v is positive).
Therefore, from the above results we conclude that:
When an object is placed beyond 2f, the image is real, inverted and diminished and is formed between f and 2f.
Answer 19:
Here, filament of the lamp acts as an object.
v = Image distance
and u = Object distance
According to the question:
v + u = 80 (taking magnitude only) …(i)
and magnification m = v/u = 3
or, v = 3 u
or, v -3u = 0 …(ii)
Solving (i) and (ii), we get:
u = 20 cm
For the focal length of the lens, we have:
Distance of lens from the filament, u = -20 cm
v = 3 × u = 3 × 20 = 60 cm
Applying lens formula, we get:
1/v – 1/u = 1/f
1/60 + 1/20 = 1/f
(1+3)/60= 1/f
1/f=4/60
f=15 cm
So, focal length f = 15 cm
Answer 20:
Given:
Image distance, v = -12 cm (Image is erect.)
Height of the object, h = 0.5 cm
Height of the image, h’ = 2.0 cm
Applying magnification formula, we get:
m = v/u = h’/h
-12/u = 2.0/0.5
or, object distance, u = -3 cm
Applying lens formula, we get:
1/v – 1/u = 1/f
1/(-12) -1/(-3) = 1/f
or, 1/f = 3/12
or, focal length, f = 4.0 cm
Answer 21:
Given:
Focal length, f = 0.10 m = 10 cm
Object distance, u = -0.08 m = -8 cm
Height of the object, h = 5 mm = 0.5 cm
Applying lens formula, we get:
1/v -1/u = 1/f
or, 1/v = 1/f + 1/u
or, 1/v = 1/10 + 1/(-8)
Image distance, v = -80/2 = -40 cm
or, v = -0.40 m
Thus, the position of image is 0.40 m from the lens on the same side as the object (on the left of lens).
Magnification, m = h’/h = v/u
m = h’/0.5 = (-40)/(-8) = + 5
or, size or height of image, h’ = 2.5 cm or 25 mm
Further, the value of magnification is positive; therefore, the image is virtual and erect.
Answer 22:
Given:
Focal length, f = 6 cm
Object distance, u = -4 cm
Size of the object, h = 0.5 cm
Applying lens formula, we get:
1/v -1/u = 1/f
or, 1/v = 1/f + 1/u
= 1/6 + 1/(-4) = -1/12
or, image distance, v = -12 cm
Applying magnification formula, we get:
m = v/u = h’/h
or, m = (-12)/(-4) = h’/0.5
or, 3 = h’/0.5
or, h, =3×0.5
Size of the image, h’ = 1.5 cm
Therefore, the height of the image is 1.5 cm. The value of magnification is positive; therefore, the image is virtual, erect and three times magnified.
Answer 23:
Given:
Focal length, f = 10 cm
Magnification, m = +4 (Image is erect.)
Object distance, u = ?
Applying magnification formula, we get:
m = v/u
or, 4 = v/u
or, v = 4u
Applying lens formula, we get:
1/v -1/u = 1/f
1/4u – 1/u = 1/10
or, u = -30/4
or, u = -7.5 cm
Thus, the object must be placed at a distance of 7.5 cm in front of the lens.
Answer 24:
Given:
m = -10 (negative sign implies real image)
f = 20 cm
Object distance = -u
Lens formula is given by:
1f=1v-1u
Magnification in case of convex lens is given by:
m =v-u
v = mu
v=(-10)×(-u) =10 u
Putting these values in lens formula, we get:
1f=1v -1u⇒120=110u+1u ⇒ 120=1+1010u⇒ 120=1110u⇒ 10u=220⇒ u=22010=22 cm
Thus, object distance = -u = -22 cm
So, the slide is placed at a distance 22 cm from the poles of the lens.
Answer 25:
Converging lens is a convex lens.
Distance of the object from the lens (u) = -4
Distance of the image from the lens (v) = 12
(a)
Magnification of the lens is given by: m=vu⇒m=12-4=-3Thus, magnifiction produced by the lens is 3.(b) Lens formula is given by: 1f=1v-1u⇒1f=112-1-4⇒1f=112+14⇒1f=1+312 ⇒ f=3 cm
(c)

Answer 26:
Converging lens is a convex lens
Given:
Focal length (f) = +8 cm
Height of the object (h) = +2
(a)
(i) Object distance (u) = -12
Lens formula is given as:
1f=1v-1u
⇒18=1v-1-12⇒18=1v+112⇒1v=18-112⇒1v=3-224⇒1v=124⇒ v=24 cm
Image is at a distance of 24 cm from the convex lens; therefore, we have:
Magnification =vu
Magnification (m) = 24-12
m = -2
Hence, the image is real and inverted.
(ii) Object distance (u) = -6
According to lens formula: 1f=1v-1u⇒18=1v-1-6⇒18=1v+16⇒18-16=1v⇒3-424=1v⇒-124=1v ⇒v=-24 cmImage is at a distance of 24 cm in front of the lens; therefore, we have: Magnification (m)=vu m=-24-6 m=4 m=hiho ⇒ m= hi2 ⇒ hi=2×4 ⇒ hi=8 cmHeight of the image is 8 cm. Here, height is positive; therefore, image is virtual and erect.
(c) The practical application for case (1) is that it can be used as a corrective lens for a farsighted person and for case (2), it can be used as a magnifying lens for reading purposes.
Answer 27:
(a) Given:
Object distance (u) = -24
Focal length (f) = +8
Object height (h) = 3
Lens formula is given by:1f=1v-1u⇒18=1v-1-24⇒18=1v+124⇒18-124=1v⇒3-124=1v⇒224=1v ⇒ v=12 cm Image will be form at a distance of 12 cm on the right side of the convex lens.Magnification m=vu ⇒ m=12-24 ⇒ m=-12So, the image is diminished. Negative value of magnification shows that the image will be real and inverted.
m=hiho-12=hi3 hi=-32 hi =-1.5 cm Height of the image will be 1.5 cm. Here, negative sign shows that the image will be in the downward direction.
(b) Object distance (u) = -3
1f=1v-1u⇒18=1v-1-3⇒18=1v+13⇒18-13=1v⇒3-824=1v⇒-524=1v⇒ v=-245⇒v=-4.8 cmThe image will be at a distance of 4.8 cm in front of the lens.Magnification (m)=vu ⇒ m=-4.8-3 ⇒ m=1.6 Positive value of magnification shows that the image is virtual and erect. m=hiho ⇒ 1.6=hi3 ⇒ hi=3×1.6 =4.8 cm Positive sign of the image shows that the image will be formed above the principal axis.
(c) Case (b) illustrates the working of magnifying lens as the object is between the focus and optical centre.
Answer 28:
(i) u = -0.50 m = -50 cm
f = 0.20 m = 20 cm
Lens formula:
1f=1v-1u⇒120=1v-1-50⇒1v=120-150⇒1v=5-2100⇒1v=3100⇒v=1003 cmThe image will be at a distance of 33.3 cm on the right side of the mirrormagnification m=vum=33.3-50m=-0.66magnification is negative therefore image will be real and inverted.
(ii) u = -25 cm
f = 20 cm
1f=1v-1u⇒120=1v-1-25⇒1v=120-125⇒1v=5-4100⇒1v=1100⇒v=100 cm The image will be formed at a distance of 100 cm behind the mirrror.Magnification, m=vu ⇒ m=100-25 ⇒ m=-4Magnification is negative, which means the image is real and inverted.
(c) u = -15 cm
f = 20
Substituting these values in the lens formula, we get:1f=1v-1u⇒120=1v-1-15⇒120=1v+115⇒120-115=1v⇒1v=3-460⇒1v=-160 v=-60 cmThe image will be formed at a distance of 60 cm in front of the mirror.m=vu⇒m=-60-15⇒m =4Magnification is positive; therefore, the image is virtual and erect.
(b)
We can use case (ii) for a film projector.
We can use case (i) for a camera.
We can use case (ii) for a magnifying glass.
Answer 29:
(a) Both concave
According to the sign convention, the focal length for both a concave mirror and a concave lens is negative.
Answer 30:
(d) Less than 1, equal to 1 or more than 1
The size of the image formed by a convex lens may be less than, equal to or greater than the size of the object .
Exercise : Solution of Questions on page Number :248
Answer 31:
(c) Less than 1:
Magnification =size of the imagesize of the object
From the formula, it is clear that the magnification varies directly with the size of the image. The image formed by a concave mirror is always smaller than the size of the object. Therefore, the magnification of a concave mirror is always less than 1.
Answer 32:
(b) Between F and 2F
In the case of a convex lens, for an object placed between F and 2F, the image formed will be real, inverted and enlarged.
Answer 33:
(c) At less than f
since the magnification is positive, the image formed is virtual, erect and enlarged. This is the case when an object is placed at a distance of less than f of the lens.
Answer 34:
(b) At 2f
When an object is placed at 2f, the size of the image formed will be equal to the size of the object. Therefore, we get a magnification of 1.
Answer 35:
(d) Beyond 2F: since the magnification is negative and less than 1, the image formed is real, inverted and diminished. Therefore, the object should be placed beyond 2F.
Answer 36:
(c) 4.0
Magnification is given by:

Answer 37:
(b) Between 10 cm and 20 cm:
the magnification is -2, which means that the image is real, inverted and magnified. A convex mirror forms a real, inverted and magnified image when an object is placed between F and 2F .
Answer 38:
(c) At less than 15 cm:
A convex lens forms a virtual, erect and magnified image when an object is placed within the focus.
Answer 39:
(b) At 24 cm
For an object placed at a distance of 2F from a convex lens, the size of the image so formed is equal to the size of the object.
Answer 40:
(c) Beyond 16 cm
For an object placed beyond 2f of a convex lens, the image formed is real, inverted and smaller than the object.
Answer 41:
(a) Since the focal length is a constant quantity, we have to pair the object distance(u) and the image distance (v) such that the focal length always comes out to be the same. From the above argument, we get the correct order of the image distance as 100 , 60 , 40 , 30 and 24. The reason being, as the object is carried far from a convex lens, the image is formed closer to the lens.
(b) Lens formula is given by:
 mean
mean
The image distance will be 25.7 cm if the object distance is 90 cm.
(c) The object distance of 25 gives the biggest image because at this position, the object is between f and 2f . We know that when an object is placed between f and 2f of a convex lens, we get a real, inverted and magnified image.
(d) The image of an object at 2f is formed at 2f. This means that the pair of u and v that is equal in value gives us the value of 2f, which is 40. Hence, the value of f (focal length) is 40/2.
∴ f=20 cm.
Answer 42:
(a) Given, focal length (f) = 100 mm
Size of object (h) = 16 mm
Image distance (v) = 25 cm = 250 mm

The object is at a distance of 16.66 cm and in front of the mirror. The image is at a distance of 25 cm and in front of the mirror. Therefore, the distance between the object and the image is 25 cm-16.66 cm = 7.14 cm.
(b) The object should be placed at the focus for the image to be formed at infinity.
Answer 43:
Given,
Height of image ( h’) = – 3 cm (negative because image is real)
Height of object = 1 cm
Let us first define the sign convention:
Since the object distance is always negative, let it be -u.
Since the focal length is positive for a convex lens, let it be +f .
 mean
mean
(i) Since object distance (u) is always negative, and the ratio![]() here is negative, v must be positive, i.e., it is on the right side of the lens.
here is negative, v must be positive, i.e., it is on the right side of the lens.
Now, we have object distance + image distance = 15
v + (-u) = 15 (using sign convention)mean


Exercise : Solution of Questions on page Number :249
Answer 44:
Height of object (h) = 50 cm
Height of image (h’) = -20 cm (real and inverted)
Distance of image from the lens (v) = 10 cm
Distance of object from the lens (u) = ?
Focal length of the lens (f)= ?
We know, magnification (m) of the lens is given by:
![]() mean
mean
Thus, substituting the values of v, h and h’, we get:

Using the lens formula:

Exercise : Solution of Questions on page Number :251
Answer 1:
It is a concave lens because only a concave lens forms a diminished and erect image of the object at all times.
Answer 2:
The rays will diverge and appear to meet at the focus of the lenmeans.

Exercise : Solution of Questions on page Number :252
Answer 3:
(a) A concave lens is thinner in the middle than at the edges.
(b) A convex lens is thicker in the middle than at the edges.
Answer 4:
A ray of light passing through the focus of a concave lens will become parallel to the principal axis after refraction.

Answer 5:
(a) A convex lens can form two types of images: (i) real and inverted and (ii) virtual and erect. Real and inverted images are formed when an object is placed beyond the focus of the lens and virtual and erect images are formed when an object is placed between the focus and the optical centre.
(b) A concave lens always forms a virtual and erect image.
Answer 6:
The complete path of the light ray is as follows:

Answer 7:
(a) A convex lens converges rays of light, whereas a concave lens diverges rays of light.
(b) Lenses refract light to form images: a converging lens can form both real and virtual images, but a diverging lens forms only virtual images.
Answer 8:
It is a concave lens because only a concave lens forms a diminished image at all times.
Answer 9:
For a concave lens, when the object lies anywhere between the optical center (C) and infinity, the image is formed between the optical center (C) and the focus. Therefore, when the object is placed at 2f from the concave lens of focal length f, the image is formed between the optical center (C) and the focus (f). Also, the image formed is virtual, erect and diminished. The following ray diagram illustrates the image formed by the concave lens:

Answer 10:
The image formed by a concave lens is always virtual because it is formed on the left side of the lens. Let us take an object AB beyond the focus (f) as shown in the figure. The image A’B’ is formed on the left side of the concave lens and is therefore, virtual. The image is upright. Also the height of A’B’ is less than the height of AB. Therefore, the image is diminished.
 mean
mean
Diagram to show the formation of image by a concave lens.mean
Answer 11:
(a) In the case of a concave lens, when an object is placed anywhere between the optical centre and infinity, the image is formed between the optical centre and the focus. The image formed is virtual, erect and diminished.
(b) In the case of a concave lens, when an object is placed at infinity, the image is formed at the focus. The image formed is virtual, erect and highly diminished.
Answer 12:
(a) A lens that converges a parallel beam of light passing through it to a point is known as a converging lens. The convex lens is a converging lens. The ray diagram below shows the parallel light rays converging at the focus of a convex lens.
 mean
mean
(b) The lens which diverges a parallel beam of light passing through it is known as a diverging lens. A concave lens is a diverging lens. The ray diagram below shows the parallel light rays getting diverged when passed through a concave lens.
 mean
mean
Answer 13:
For an object placed anywhere in front of a concave lens, the image so formed is always virtual, erect and diminished in size. The position of the image is between the optic centre and the focus of the lens, as shown in the figure

Answer 14:
(a) When a pencil is seen through a concave lens placed close to it, it appears smaller than it’s actual size. Because a concave lens always produces a virtual image, the image formed is virtual.
(b) When a pencil is seen through a convex lens placed close to it, the image so formed appears larger than it’s actual size. The reason for this is, the pencil is placed within the focus of the lens and therefore, the image formed is virtual.
Answer 15:
(a)(i) When an object is placed at 2F’ of a converging lens, the image fomeanrmed is real, inverted and of same size as the object. The image position at 2F is shown in the figure:

(a)(ii) When an object is placed beyond F of a diverging lens, the image formed is virtual, erect and diminished. The position between the focus and the optic centre is as shown in the figure:
 mean
mean
(b)
A convex lens is used as a magnifying glass.
A concave mirror is used as a shaving mirror.
A convex mirror is used as rear view mirror in vehicles.
A concave lens is used for correcting myopia.
Answer 16:
(a)(i) When an object is placed between the optic centre and the focus of a converging lens, the image formed is virtual, erect and magnified as shown in the figure.
 mean
mean
(a)(ii) When an object is placed anywhere between the optic centre and infinity of a converging lens, the image formed is virtual, erect, and diminished as shown in the figure.

| S.No | Virtual image made by a converging lens | Virtual image made by a diverging lens |
| 1 | Image is larger than the object | Image is smaller than the object |
Answer 17:
(c) Spectacles for the correction of short sight
A diverging lens is used in spectacles to correct short-sightedness.
Answer 18:
(c) virtual, erect and diminished
A concave lens always forms a virtual, erect and diminished image.
Answer 19:
(c) either a concave mirror or a convex lens
Both a concave mirror and a convex lens focus parallel light beams coming from a distant object onto the focus.
Exercise : Solution of Questions on page Number :253
Answer 20:
(c) a convex lens
The beam of light is converging when it is coming out of the box; therefore, there should be a convex lens inside the box.
Answer 21:
(b) a concave lens
This is because the emergent rays of light are diverging.
Answer 22:
(c) a concave lens
A concave lens always forms a virtual image, smaller than the size of the object.
Answer 23:
(a) The focal length of lens A is 10 cm as only a converging lens forms a real, inverted, magnified image at great distance when object is placed at the focus of the lens.
(b) The focal length of lens B is 5 cm as only a converging lens forms a real, inverted and same size image of object when the object is placed at 2F position of the lens.
(c) Lens A is a converging (convex) lens as only a converging lens forms a real image.
(d) Lens B is a converging (convex) lens as only a converging lens forms a real image.
Answer 24:
When the fork is seen through lens A, it appears to be diminished. Such diminished object is observed when it is placed near a concave lens. Therefore, lens A is concave, i.e., diverging in nature.
When the fork is seen through lens B, it appears to be enlarged. Such enlarged image is formed by a convex lens when an object is placed between the lens and its focus. Therefore, lens B is a convex, i.e., converging in nature.
Answer 25:
(a) A convex lens can form an inverted magnified image.
(b) A convex lens can form an erect magnified image.
(c) A convex lens can form an inverted diminished image.
(d) A concave lens can form an erect diminished image.
Exercise : Solution of Questions on page Number :255
Answer 1:
(a) Negative magnification shows that the image is real and inverted. Therefore, it’s a convex lens.
(b) Positive magnification shows that image is virtual and erect. The lens can be concave or convex in nature. However, a convex lens always forms a magnified virtual image. According to the given magnification, the size of the image is smaller than the size of the object. Therefore, the lens must be concave in nature.
Answer 2:
Since the object is at infinity, the image will be formed at the focus. The image distance will be equal to the focal length of the lens. Therefore, the focal length of the lens is 20 cm.
Exercise : Solution of Questions on page Number :256
Answer 3:
Object distance u = -4 cm (left side of the lens)
Focal length f = -12 cm (left side of the lens)
Image distance v = ?
From the lens formula, we know that:
1v-1u=1f
Substituting the values of v and u, we get:
1v-1u=1f⇒1v-1-4=1-12⇒1v=-112-14⇒1v=-3-112⇒1v=-412⇒v=12-4⇒v=-3 cm
Thus, the image is virtual and formed 3 cm away from the lens, on the left side.
Answer 4:
Focal length f = -15 cm (negative due to sign convention)
Image distance v = – 10 cm (negative due to sign convention)
Object distance u = ?
Applying the lens formula:
1v-1u=1f
⇒ 1-10-1u=115⇒ 1u=115-110⇒ 1u=2-330⇒ 1u=-130 ∴ u=-30 cm.
The object should be place at a distance of 30 cm from the concave lens, and on the left side.

Answer 5:
Object distance u = – 60 cm (As the object is on the left side of the lens)
Image distance v = – 20 cm (As the image is virtual)
Magnification m = vu = -20-60 = 13
Because the image is diminished and virtual, the lens is concave and diverging in nature.
Using the lens formula:
1f=1v-1u⇒1f=1-20-1-60⇒1f=1-20+160⇒1f=-3+160=-130
∴ f = – 30 cm.
Therefore, the focal length of the lens is 30 cm.
The lens is diverging in nature because a negative focal length indicates a concave lens .
Answer 6:
Focal length of concave lens f = – 20 cm (focal length is negative for a concave lens)
Image distance v = – 15 cm ( negative sign is given because the image formed by a concave lens is on the left side of the lens)
Using the lens formula:
1f=1v-1u⇒1-20=1-15-1u⇒1u=120+1(-15)⇒1u=3-460=-160
∴ u = – 60 cm.
The negative sign shows that the object is on the left side of the concave lens.
Therefore, the object is at a distance of 60 cm and on the left side of the concave lens.
Answer 7:
Focal length of concave lens f = – 15 cm
Image distance v = – 10 cm ( image formed by a concave lens is on the left side of the lens)
Using the lens formula:
1f=1v-1u⇒1-15=1-10-1u⇒1u=115-110 ⇒ 1u =2-330=-130
∴ u = – 30 cm.
The object is at a distance of 30 cm from the lens and on its left.
The magnification of the concave lens is given as:
Magnification=image distanceobject distance⇒m =vu=-10-30=13
Since the magnification is less than 1, the image is smaller than the object.
Answer 8:
Focal length of concave lens f = – 0.30 m
Object distance u = – 0.20 m
Height of object h1 = 12 mm = 0.012 m
Height of image h2 =?
Using the lens formula:
1f=1v-1u⇒1-0.30=1v-1(-0.20)⇒1v=1-0.30-10.20⇒1v=-2-30.60=-10.12
∴ v = – 0.12 m = – 12 cm.
The negative sign indicates that the image is formed on the left side of the lens. Therefore, the image is virtual.
Magnification is given as:
Magnification=image distanceobject distance=height of imageheight of object⇒vu=h2h1⇒-0.12-0.20=h20.012⇒h2=0.12×0.0120.20=0.0072 m =+ 7.2 mm
Here, the positive sign shows that the image is erect. Also, the size of the image is smaller than the size of the object and is diminished.
Therefore, the image formed by the concave lens is virtual, diminished and erect.
Answer 9:
Focal length of the concave lens, f = – 20 cm
Image distance from the concave lens, v = – 15 cm (image formed by a concave lens is on the left side of the lens)
Height of the object, h1 = 5 cm
Height of the image = h2
Using the lens formula:
1f=1v-1u⇒1-20=1-15-1u⇒1u=120-115⇒1u=3-460=-160
∴ u = – 60 cm.
Therefore, the object is at a distance of 60 cm from the concave lens and on the left side of it.
The magnification of the concave lens is given as:
Magnification=image distanceobject distance=height of imageheight of object⇒vu=h2h1⇒-15-60=h25⇒h2=54=1.25 cm.
Therefore, the size of the image is 1.25 cm.
Answer 10:
(a) Focal length of the converging lens, i.e., convex lens f = + 15 cm
Object distance u = – 20 cm
Using the lens formula:
1f=1v-1u⇒115=1v-1-20⇒ 115=1v+120⇒1v=115-120⇒1v=4-360=160
∴ v = +60 cm.
Therefore, the image formed is real. It is at a distance of 60 cm from the lens and to its right.
Magnification=image distanceobject distance∴m =vu=60-20=-3.
The magnification is greater than 1. Therefore, the image is magnified.
The negative sign shows that the image is inverted.
(b) Focal length of the diverging lens, i.e., concave lens f = – 15 cm
Object distance u = – 20 cm
Using the lens formula:
1f=1v-1u⇒1-15=1v-1-20⇒1v=1-15-120⇒1v=-4-360=-760
∴ v = – 8.57 cm.
Therefore, the image formed is virtual. It is at a distance of 8.57 cm from the lens and to its left.
Magnification=image distanceobject distance∴m =vu=-8.57-20=+0.42.
The magnification is less than 1. Therefore, the image is diminished. The positive sign indicates that the image is erect.
Answer 11:
A concave lens is also known as a diverging lens.
Focal length of concave lens, f = -15 cm
Object distance from the lens, u = -40 cm
Height of the object, h1 = 2.0 cm
Height of the image, h2 = ?
Using the lens formula, we get:
1f=1v-1u⇒1-15=1v-1-40 ⇒1-15=1v+140⇒1v=1-15-140⇒1v=-8-3120⇒1v=-11120
∴ v = – 10.90 cm
Therefore, the image is formed at a distance of 10.90 cm and to the left of the lens.
Magnification of the lens:
Magnification=Image distanceObject distance=Height of the imageHeight of the object⇒vu=h2h1⇒-10.90-40=h22⇒h2=+0.54 cm
The height of the image formed is 0.54 cm. Also, the positive sign of the height of the image shows that the image is erect.
Answer 12:
(a) Object height (h) = 2 cm
Image height (h’) =?
Object distance (u) = -20 cm (sign convention)
(i) Focal length (f) = -40 cm (sign convention)
Image distance (v) = ?
Applying the lens formula:
1v-1u=1f⇒ 1v-1-20=1-40 ⇒ 1v+120=-140 ⇒ 1v=-140-120⇒ 1v=-1-240⇒ 1v=-340 ∴v =-403=-13.33 cm.
Negative sign shows that the image formed is virtual and erect.
Now, magnification m = vu=h’h
h’=vux h h’=-13.33-20x 2=1.33 cm
Height of the image is 1.33 cm.
(ii) Focal length (f) = 40 cm (sign convention)
Image distance (v) = ?
Applying the lens formula:
1v-1u=1f⇒ 1v-1-20=140 ⇒ 1v+120=140 ⇒ 1v=140-120⇒ 1v=1-240⇒ 1v=-140 ∴ v =-40 cm.
Negative sign shows that the image formed is virtual and erect.
Now, magnification = vu=h’h
h’=vux h h’=-40-20x 2=4 cm.


Answer 13:
(a)(i) The image formed by the lens is virtual, erect and diminished in size.

(a)(ii) Object distance (u) = -150 mm = -15 cm (sign convention)
Focal length (f) = -100 mm = -10 cm (sign convention)
Image distance (v) = ?
Applying the lens formula, we have:

(b)
| S.No | Image formed by a diverging lens | Image formed by a converging lens |
| 1 | Image formed is virtual and erect | Image formed is real and inverted |
| 2 | Image is smaller in size than the object | Image is larger in size than the object |
Answer 14:
(c) 60 cm
Object distance from the lens, u = -30 cm
Image distance from the lens, v = -20 cm
Using the lens formula, we get:

Therefore, the focal length of the lens is 60 cm
Answer 15:
(d) image distance is always negative
Because a concave lens always forms a virtual image on the left side of the lens.
Answer 16:
(d) The mirror is convex but the lens is concave
Because both convex mirror and concave lens have positive magnification.
Answer 17:
(c) The lens is convex but the mirror is concave.
Because both concave mirror and convex lens have positive magnification.
Exercise : Solution of Questions on page Number :257
Answer 18:
(a)
Given:
Focal length, f = 50 mm = 5 cm
Object distance, u = -20 cm
Image distance, v = ?
Putting these values in lens formula, we get:
1v-1u=1f⇒ 1v-1-20=15⇒ 1v+120=15⇒ 1v=15-120
⇒1v=20-5100⇒1v=15100⇒v=203⇒v=6.66 cm
Film should be adjusted at a distance of 6.66 cm behind the lens.
(b)
Given:
Diameter of object, h = 5 cm
Diameter of image, h’ = ?
Magnification, m = h’h=vu
Therefore
h’ =vuxh
h’ = 6.6620×5=1.66 cm
(c) It is a convex lens.
Answer 19:
Given:
Object distance (u) = -2 m
Image distance (v) = ?
Magnification (m) = 0.25 (one-fourth of the size of the image)
Focal length (f) = ?
Magnification (m) = vu
0.25=v-2 v=-0.5 m
Putting these values in lens formula, we get:
1v-1u=1f⇒1-0.5-1-2=1f⇒1-0.5+12=1f ⇒1f=12-10.5 ⇒1f=12-105⇒ 1f=-32⇒ f = -0.66 m
Negative sign of focal length shows that lens is diverging in nature. Hence, it is a concave lens.
Answer 20:
(a) The image here can be taken on a screen. This means that the image is real. Further, we know that only convex lens forms a real image; therefore, a convex lens has been used here.
(b) Magnification (m) = 3
Object distance (u) = ?
Image distance (v) = ?
Focal length (f) = ?
Distance between image and object (v + u) = 80 cm
v = 80 – u
We know that:
m = vu
or 3 = 80-uu
or 3u = 80 – u
or 4u = 80
or u = 20 cm
Thus, v = 80 – u
= 80 – 20 = 60 cm
Putting these values in lens formula, we get:
1v-1u=1f⇒ 160-1-20=1f⇒1+360=1f⇒ f=604=15 cm
Exercise : Solution of Questions on page Number :261
Answer 1:
Power varies inversely with the focal length. Lens A has a small focal length and hence, more power.
Answer 2The bending of light by a convex lens depends on the power of the lens. The more the power, the more the light bends. A convex lens having a short focal length will have more power and hence, a higher refraction.
Answer 3:
The power of a lens is a physical quantity with dioptre as it’s unit.
Answer 4:
1 dioptre is the power of a lens whose focal length is 1 metre.
Answer 5:
(a) Convex lens has positive power.
(b) Concave lens has negative power.
Answer 6:
A lens with a shorter focal length will have more power because power varies inversely with focal length .
Answer 7:
The power of a lens is related to the focal length as:
Power (P) =1f,
where, f is measured in metres and P in dioptres.
Answer 8:
A thick convex lens has more power than a thin one because it has greater curvature or lesser focal length than a thin lens.
Answer 9:
Given, f = 25 cm
Power of a lens is given by:
Power (P) =1f
∴ P =125×10-2 =10025= 4 D.
Answer 10:
Power of a lens is given by:
Power (P) = 1f
∴ P =10.5=2 D.
Answer 11
Power of a lens is given by:
Power (P) = 1f
∴ P = 150×10-3 = 100050= 20 D.
Answer 12:
Power of a lens is given by:
Power (P) = 1f
∴ P = 180×10-2 = 10080 = 1.25 D.
Answer 13:
Power of a lens is given by:
Power (P) = 1f
∴ P = 13×10-2 = 1003= 33.33 D (appx.)
Answer 14:
Power of a lens is given by:
Power (P) = 1f
f=1p
f=10.2 =5 m.
Answer 15:
Power of a lens is given by:
power p=1focal length
p=1f⇒f=1p⇒ f=1-2 f=-0 .5 m
Negative sign indicates that it’s a concave lens.
Answer 16:
Positive sign indicates it is a converging lens. Therefore, the lens is convex.
Answer 17Negative sign indicates it is a diverging lens. Therefore, the lens is concave in nature.
Answer 18:
(a) Positive power indicates it is a converging lens.
(b) Power of a lens is given by:
Power (P) = 1
0.5 = 1f
f=10.5
f = 2 m
Focal length of the lens is 2 m.
Answer 19:
Power of a lens is given by:
Power (P) = 1f
f=1p
⇒f=1-1.5
∴ f = -66.67 cm.
Since the power is negative, the lens is of a diverging nature. Therefore, it’s a concave lens.
Exercise : Solution of Questions on page Number :262
Answer 20:
Power of a lens is given by:
Power (P) = 1f
f = -10 cm
∴ P = 110×10-2 = -10 D.
Negative sign indicates it is a diverging lens.
Answer 21:
Power of a lens is given by:
Power (P) =1f
∴ P = ‘1150×10-3 = 6.67 D (apprx.)
Since the power is positive, the lens is convex in nature.
Answer 22:
(a) The reciprocal of the focal length in metres gives you the power of the lens, which is measured in dioptres.
(b) For converging lenses, the power is positive while for diverging lenses, the power is negative.
Answer 23:
Object distance u = -15 cm
Height of object h = 4 cm
Power of the lens p = -10 dioptres
Height of image h’ = ?
Image distance v = ?
Focal length of the lens f = ?
We know that:
p = 1f
f=1p⇒f=1-10⇒f=-0.1 m =-10 cm
From the lens formula, we have:
1v-1u=1f
1v-1-15=1-10⇒1v+115=-110⇒1v=-115-110⇒1v=-2-330⇒1v=-530⇒1v=-16⇒v = -6 cm
Thus, the image will be formed at a distance of 6 cm and in front of the mirror.
Now, magnification m = vu=h’h
or -6-15=h’4 h’ =6 x 415 h’ = 2415∴ h’ =1.6 cm.
Answer 24:
Object height, h = 4.25 mm = 0.425 cm (1 cm = 10 mm)
Object distance, u = -10 cm
Power, P = +5 D
Focal length, f = ?
Image distance, v = ?
Image height, h’ = ?
Power, P = 1f
f = 1p=15=0.2 m = 20 cm
Using the lens formula, we get:
1v-1u=1f
⇒1v-1-10=120⇒1v+110=120⇒1v=120-110⇒1v=-120∴ v =-20 cm
Now, magnification, m = h’h=vu
Substituting the values in the above equation, we get:
h’0.425=-20-10h’=2 x 0.425 =0.85 cm = 8.5 mm
Thus, the image is 8.5 mm long; it is also erect and virtual.
Answer 25:
(a) Power of convex lens P1 = + 5 D
Power of concave lens P2 = – 7.5 D
The combined power is the algebraic sum of the individual powers of the lenses.
∴ Power of the combination P = P1 + P2
P = 5 D – 7.5 D = – 2.5 D
Therefore, the power of the combination of the lenses is – 2.5 D.
(b) The power and focal length are related as:
P = 1f-2.5=1f∴f=-12.5 = -0.4 m = – 40 cm.
Therefore, the focal length of the combination of the lenses is 40 cm. The negative sign shows that the combination of the two lenses causes it to behave like a concave lens.
Answer 26:
Focal length of convex lens f1 = + 25 cm = + 0.25 m
Power of convex lens P1=1f1=10.25=4 D
Focal length of concave lens f2 = – 10 cm = – 0.10 m
Power of concave lens P2=1f2=1-0.10=-10 D
(a) The power of the combination of lenses is the algebraic sum of the powers of the individual lenses.
∴ Power of combination P = P1 + P2
⇒ P = 4 – 10 = – 6 D.
(b) Suppose, the focal length of the combination of the lenses is f.
The power of a lens and the focal length are related as:
P=1f-6=1ff=1-6=-0.167 m = – 16.7 cm\
Therefore, the focal length of the combination of the lenses is – 16.7 cm.
(c) The focal length of the combination of the lenses is – 16.7 cm. Here, the negative sign shows that the combination of the two lenses acts like a concave lens. Therefore, this combination of lenses is diverging.
Answer 27:
(a) The focal length of lens X, ie., f1 = 15 cm = 0.15 m
Power of lens X, P1=1f1=10.15=6.67 D
Let the power and the focal length of the lens Y be P2 and f2, respectively.
The resultant power of the combination of two lenses is the algebraic sum of the powers of the individual lenses.
∴ Power of combination of lenses P = P1 + P2
⇒ 5 = 6.67 + P2
⇒ P2 = 5 – 6.67 = – 1.67 D
The power of the lens is related to the focal length of the lens as:
P2=1f2⇒-1.67 =1f2⇒f2=1-1.67=-0.60 m = -60 cm
The focal length of the lens Y is -60 cm.
(b) The focal length of the lens Y is negative. Therefore, the lens is concave and diverging in nature.
Answer 28:
(a) The focal length of lens A is +20 cm. The positive sign indicates that lens A is convex, i.e., a converging lens.
The focal length of lens B is -10 cm. The negative sign indicates that lens B is concave, i.e., a diverging lens.
(b) Focal length of lens A, fA = +20 cm = +0.20 m
![]()
Focal length of lens B, fB = -10 cm = -0.10 m
![]()
(c) When lenses are combined, the power of the combination is the algebraic sum of the powers of the individual lenses.
∴ Power of combination of lens A and B, P = PA + PB
= 5 D – 10 D = – 5 D.
Answer 29:
(a) The power of a lens is a measure of the degree of convergence or divergence of light rays falling on it. It is defined as the reciprocal of the focal length of a lens in metres.
![]()
Therefore, the power of a lens depends on the focal length.
(b) The unit for the power of a lens is a dioptre (D). One dioptre is the power of a lens whose focal length is 1 metre.
(c) Focal length (f1) of the 1st converging lens = 20 cm = 0.2 m
Focal length (f2) of the 2nd converging lens = 40 cm = 0.4 m
Focal length (f3) of the diverging lens = -50 cm = -0.5 m (negative sign due to sign convention)
![]()


![]()
Answer 30:
(a) Power of lens A = +2D
Power of lens B = -4D

![]()
![]()
Therefore, lens A is a convex lens as it has a positive focal length and lens B is concave as it has a negative focal length.
(b)
Object distance (u) = -100 cm (sign convention)
For lens A:
Image distance (v) = ?
Using the lens formula, we get:

Negative sign shows that the image formed is virtual.
![]()
For lens B:
Image distance (v2) =?
Using the lens formula, we get:

Negative sign shows that the image formed is virtual.
∴ Magnification = vu=-20-100=-0.2.
Answer 31:
(c) R
![]()
Therefore, a lens with a small focal length will have more power.
Answer 32:
(c) +2.0 D
![]()
Answer 33:
(d) -10.0D,
![]()
Answer 34:
(b) 50 cm
![]()
Answer 35:
(c) -4 m
![]()
Exercise : Solution of Questions on page Number :263
Answer 36:
(a) -4 D
The powers of the lenses add up when brought in contact.
So, net power = +6 D + (-10 D) = -4 D.
Answer 37:
(a) 1.5D
Because power of lens adds up when placed in combination.
Therefore
Net power = 4.5 D + (-3.0 D) = 1.5 D
Answer 38:
(b) +20 cm
We know that:
![]()
Therefore

We know that power of lens adds up when lenses comes in contact. Therefore, we have:
Net power (P) = P1 + P2
Net power (P) = 10 D – 5 D = 5 D
![]()
Answer 39:
(a) These lens are thinner at the middle because they are concave lens. Further, concave lens has negative power.
(b) Lens for right eye has greater focal length because power is inversely proportional to focal length.
(c) Left eye is weaker because it has a correction with a lens of lower focal length.
Answer 40:
(a) These lenses are thicker in the middle because they are convex lens.
(b) Power is inversely proportional to focal length; therefore, shorter focal length will mean strong bending. Hence, lens for left eye will bend light rays more strongly.
(c) These spectacles will converge light rays because convex lens has positive power.
