Exercise : Solution of Questions on page Number : 130
Answer: 1
No. Work done in lifting the box increases the potential energy of the box. During lifting at every point, the force applied by us on the box in the upward direction is equal to the gravitational force acting on the box in the downward direction. Therefore, there is no change in the velocity of the box. As a result, the kinetic energy of the box will not change.
Answer: 2
No, the kinetic energy of the particle at the bottom of the inclined plane does not depend on the angle of inclination. When the particle reaches the ground, all its potential energy, while at the top of the inclined plane, is converted into kinetic energy. As we know that kinetic energy depends only on the height of the particle, it will be the same for different angles of inclination.
No, we do not need any other information to answer this question.
Answer: 3
Yes. Let us consider a block A which is resting on another block B. Block B is resting on a smooth horizontal surface. Let the coefficient of friction between the blocks be μ.
When a force F is applied on block B in the forward direction as shown in the above figure, block A moves with block B in the direction of the applied force. The frictional force on block A and the displacement will be in the forward direction. Therefore, work done by the frictional force is positive.
If we consider the reference frame of block B, then displacement of block A will be zero. Therefore, work done by the frictional force is zero.
Answer: 4
Yes. Let us consider a block A which is resting on another block B. Block B is resting on a smooth horizontal surface. Let the coefficient of kinetic friction between the blocks be μk.
When a force F is applied on block B in the forward direction as shown in the above figure, block A moves with block B in the direction of the applied force. The friction force on block A and the displacement will be in the forward direction. Therefore, work done by the friction force is positive. In this case, block A will remain in contact with block B. This shows that static friction is doing a nonzero work on an object.
Answer: 5
Yes. Let us consider an elevator accelerating upward with a body placed in it. In this case, the normal reaction offered by the floor of the elevator on the body is greater than the weight of the body acting in the downward direction. If a person is observing this from the ground, then, for him, the normal reaction is doing a positive work, as the elevator is moving upward.
Answer: 6
Yes. Let us consider an isolated system of two particles falling towards each other under their mutual gravitational force of attraction. Here, the net force on the system is zero, but the velocities of the particles keep on increasing. Also, the kinetic energy of the system is increased without applying any external force on it.
Answer: 7
In an non-inertial frame, pseudo force also comes into account. As we know that pseudo force does not exist, work-energy theorem is not valid in non-inertial frames.
Answer: 8
(i) No. As the surface is smooth and the friction is zero, work done by the force will only depend on the force and the displacement.
(ii) No, because gravitational force is a conservative force and work done by a conservative force will depend only on the force and the displacement.
Answer: 9
No, both are correct. We measure potential energy from a reference level chosen by the observer. Therefore, in this case, both observers are measuring the potential energy from different reference levels.
Answer: 10
Yes, one of them is necessarily wrong. We measure potential energy from a reference level chosen by the observer. However, the change in potential energy of a body does not depend on the level of reference.
Answer: 11
(i) During compression, the work done on the ball is positive as the direction of the force applied by the fingers is along the compression of the ball.
(ii) During expansion, the work done is negative as expansion takes place against the force applied by the fingers on the ball.
Answer: 12
(a) Work by the winning team on the losing team is positive, as the displacement of the losing team is along the force applied by the winning team.
(b) Work by the losing team on the winning team is negative, as the displacement of the winning team is opposite to the force applied by losing team.
(c) Work by the ground on the winning team is positive.
(d) Work by the ground on the losing team is negative.
(e) Total external work on the two teams is positive.
Exercise : Solution of Questions on page Number : 131
Answer: 13
When an apple falls from a tree, its gravitational potential energy decreases as it reaches the ground. After it strikes the ground, its potential energy will remain unchanged.
Answer: 14
When a person pushes his bicycle up on an inclined plane, the potential energies of the bicycle and the person increase because moving up on the inclined plane the kinetic energy decreases. and as mechanical energy is sum of kinetic energy and potential energy, and remains constant for a conservative system. Therefore, potential energy must increase in this case.
Answer: 15
The magnetic force on a charged particle is always perpendicular to its velocity. Therefore, the work done by the magnetic force on the charged particle is zero. Here, the kinetic energy and speed of the particle remain unaffected, while the velocity changes due to the change in direction of its motion.
Answer: 16
(a)
Initial kinetic energy of the ball, Ki=12mv2
Here, m is the mass of the ball.
The final kinetic of the ball is zero.
(b)
Work done by the kinetic friction is equal to the change in kinetic energy of the ball.
∴ Work done by the kinetic friction = Kf-Ki=0-12mv2
= -12mv2
Answer: 17
The relative velocity of the ball w.r.t. the moving frame is given by vr=v-v0.
(a) Initial kinetic energy of the ball = 12mvr2=12m(v-v0)2
Also, final kinetic energy of the ball = 12m(0-v0)2=12mv02
(b) Work done by the kinetic friction = final kinetic energy – initial kinetic energy
= 12m(v0)2-12m(v-v0)2
= -12mv2+mvv0
Answer: 1
(d) the speed does not depend on the initial direction
As the stone falls under the gravitational force, which is a conservative force, the total energy of the stone remains the same at every point during its motion.
From the conservation of energy, we have:
Initial energy of the stone = final energy of the stone
i.e., (K.E.)i+(P.E.)i=(K.E.)f+(P.E.)f
⇒ 12mv2+mgh=12m(vmax)2⇒vmax=v2+2gh
From the above expression, we can say that the maximum speed with which stone hits the ground does not depend on the initial direction.
Answer: 2
(b) 2E
Let xA and xB be the extensions produced in springs A and B, respectively.
Restoring force on spring A, F=kAxA …(i)
Restoring force on spring B, F=kBxB …(ii)
From (i) and (ii), we get:
kAxA=kBxB
It is given that kA = 2kB
∴ x B = 2xA
Energy stored in spring A:
E=12kAxA2 …(iii)
Energy stored in spring B:
E’=12kBxB2=12(kA2)(2xA)2
∴E’=2×12kAxA2=2E [From (iii)]
Answer: 3
(d) -14kx2
The work done by the spring on both the masses is equal to the negative of the increase in the elastic potential energy of the spring.
The elastic potential energy of the spring is given by Ep=12kx2.
Work done by the spring on both the masses = -12kx2
∴ Work done by the spring on each mass = 12-12kx2=-14kx2
Answer: 4
(c) potential energy
The negative of the work done by the conservative internal forces on a system is equal to the changes in potential energy.
i.e. W=-∆P.E.
Answer: 5
(a) total energy
When work is done by an external forces on a system, the total energy of the system will change.
Answer: 6
(a) total energy
The work done by all the forces (external and internal) on a system is equal to the change in the total energy.
Answer: 7
(c) Potential energy
The potential energy of a two particle system depends only on the separation between the particles.
Answer: 8
(c) mgvt sin2θ
Distance (d) travelled by the elevator in time t = vt
The block is not sliding on the wedge.
Then friction force (f) = mg sinθ
Work done by the friction force on the block in time t is given by
W=Fdcos(90-θ)⇒W=mgsinθ×d×cos(90-θ)⇒W=mgdsin2θ∴W=mgvtsin2θ
Answer: 9
(d) none of these.
The net force on the block is not zero, therefore the block will not be in any given equilibrium.
Answer: 10
(c) 3gl
Suppose that one end of an extensible string is attached to a mass m, while the other end is fixed. The mass moves with a velocity v in a vertical circle of radius R. At some instant, the string makes an angle θ with the vertical as shown in the figure.
For a complete circle, the minimum velocity at L must be vL=5gl.
Applying the law of conservation of energy, we have:
Total energy at M = total energy at L
i.e., 12mvM2+mgl=12mvL2⇒12mvM2=12mvL2-mglUsing vL≥5gl,
we have : 12mvM2≥12m(5gl)-mgl∴vM=3gl
Exercise : Solution of Questions on page Number : 132
Answer: 1
(a) must depend on the speed of projection
(b) must be larger than the speed of projection
Consider that the stone is projected with initial speed v.
As the stone is falls under the gravitational force which is a conservative force, the total energy of the stone remains the same at every point during its motion.
From the conservation of energy, we have:
Initial energy of the stone = final energy of the stone
i.e., (K.E.)i+(P.E.)i=(K.E.)f+(P.E.)f
⇒12mv2+mgh=12m(vmax)2⇒vmax=v2+2gh
From the above expression, we can say that the maximum speed with which the stone hits the ground depends on the speed of projection and greater than it.
Answer: 2
(a) always
According to the work-energy theorem, the total work done on a particle is equal to the change in kinetic energy of the particle.
Answer: 3
(c) its kinetic energy is constant
(d) it moves in a circular path.
When the force on a particle is always perpendicular to its velocity, the work done by the force on the particle is zero, as the angle between the force and velocity is 90°. So, kinetic energy of the particle will remain constant. The force acting perpendicular to the velocity of the particle provides centripetal acceleration that causes the particle to move in a circular path.
Answer: 4
(d) acceleration of the block
Acceleration of the block will be the same to both the observers. The respective kinetic energies of the observers are different, because the block appears to be moving with different velocities to both the observers. Work done by the friction and the total work done on the block are also different to the observers.
Answer: 5
(a) the path taken by the suitcase
(b) the time taken by you in doing so
(d) your weight
Work done by us on the suitcase is equal to the change in potential energy of the suitcase.
i.e., W = mgh
Here, mg is the weight of the suitcase and h is height of the table.
Hence, work done by the conservative (gravitational) force does not depend on the path.
Answer: 6
(a) the force is always perpendicular to its velocity
(c) the object is stationary but the point of application of the force moves on the object
(d) the object moves in such a way that the point of application of the force remains fixed.
No work is done by a force on an object if the force is always perpendicular to its velocity. Acceleration does not always provide the direction of motion, so we cannot say that no work is done by a force on an object if it is always perpendicular to the acceleration. Work done is zero when the displacement is zero.
In a circular motion, force provides the centripetal acceleration. The angle between this force and the displacement is 90°, so work done by the force on an object is zero.
Answer: 7
(a) The string becomes slack when the particle reaches its highest point.
(d) The particle again passes through the initial position.
The string becomes slack when the particle reaches its highest point. This is because at the highest point, the tension in the string is minimum. At this point, potential energy of the particle is maximum, while its kinetic energy is minimum. From the law of conservation of energy, we can see that the particle again passes through the initial position where its potential energy is minimum and its kinetic energy is maximum.
Answer: 8
(b) The resultant force on the particle must be at an angle less than 90° with the velocity all the time.
(d) The magnitude of its linear momentum is increasing continuously.
Kinetic energy of a particle is directly proportional to the square of its velocity. The resultant force on the particle must be at an angle less than 90° with the velocity all the time so that the velocity or kinetic energy of the particle keeps on increasing.
The kinetic energy is also directly proportional to the square of its momentum, therefore it continuously increases with the increase in momentum of the particle.
Answer: 9
(a) at spring was initially compressed by a distance x and was finally in its natural length
(b) it was initially stretched by a distance x and and finally was in its natural length
For an elastic spring, the work done is equal to the negative of the change in its potential energy.
When the spring was initially compressed or stretched by a distance x, its potential energy is given by
P.E.i=12kx2.
When it finally comes to its natural length, its potential energy is given by
P.E.f=0.
∴ Work done = -P.E.f-P.E.i=-0-12kx2=12kx2
Answer: 10
(b) The tension in the string is F.
Tension in the string is equal to F, as tension on both sides of a friction less and mass less pulley is the same.
i.e., T – Mg = Ma
⇒ T = Mg + Ma
So, the tension in the string cannot be equal to Mg.
The change in kinetic energy of the block is equal to the work done by gravity.
Hence, the work done by gravity is 20 J in 1 s, while the the work done by the tension force is zero.
Answer: 1
Total mass of the system (cyclist and bike), M=mc+mb=90 kg
Initial velocity of the system, u=6.0 km/h=1.666 m/sec
Final velocity of the system, ν=12 km/h=3.333 m/sec
From work-energy theorem, we have:
Increase in K.E.=12Mν2-12mu2=1290×3.3332-12×90×1.662=499.4-124.6=374.8=375 J
Answer: 2
Mass of the block, Mb=2 kg Initial speed of the block, u=10 m/s Also, a=3 m/s 2 and t=5 Using the equation of the motion,
we have : ν=u+at =10+3×5=25 m/s
∴ Final K.E.=12mν2 =12×2×625=625 J
Answer: 3
Resisting force acting on the box, F=100 N
Displacement of the box, S = 4 m
Also, θ=180°
∴ Work done by the resisting force, W=→F·→S=100×4×cos180°=-400 J
Answer: 4
Mass of the block, M=5 kg Angle of inclination, θ=30° Gravitational force acting on the block, F=mg
Work done by the force of gravity depends only on the height of the object, not on the path length covered by the object.
Height of the object, h=10×sin30° =10×12=5 m
∴ Work done by the force of gravity, w=mgh =5×9.8×5=245 J
Answer: 5
Given:
F=2.50 N, S=2.5 m and m=15 g=0.015 kg
Work done by the force,
W=F·S cos 0° acting along the same line=2.5×2.5=6.25 J
Acceleration of the particle is,
a=Fm=2.50.015=5003 m/s2
Applying the work-energy principle for finding the final velocity of the particle,
12mv2-0=6.25⇒ ν=6.25×20.15=28.86 m/s
So, time taken by the particle to cover 2.5 m distance,
t=ν-uα=28.86×3500
∴ Average power=Wt=6.25×50028.86×3=36.1 W
Answer: 6
Initial position vector,
r1→=2i→+3j→
Final position vector,
r→2=3i→+2j→
So, displacement vector,
r→=r→2-r→1=3i→+2j→-2i→+j→=i→-j→Force acting on the particle, F→=5i→+5j→So,
work done=F→·S→=5×1+5 -1=0
Answer: 7
Given:
Mass of the block, m=2kg Distance covered by the man,s=40 m Acceleration of the man, a=0.5m/s2
So, force applied by the man on the box,
F=ma=2×0.5=1 N Work done by the man on the block, W=F·S=1×40=40 J
Exercise : Solution of Questions on page Number : 133
Answer: 8
Given that force is a function of displacement, i.e. F=a+bx,
where a and b are constants.
So, work done by this force during the displacement x = 0 to x = d,
W=∫0dF dxW=∫0d a+bx
dxW=ax+bx220dW=ad+bd22⇒W=a+bd2d
Answer: 9
Given: m=250 g, θ=37°, d=1 m
Here, R is the normal reaction of the block.
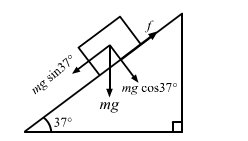
As the block is moving with uniform speed,
f=mgsin37°
So, work done against the force of friction,
W=fdcos0°W=(mgsin37°)×dW=(0.25×9.8×sin37°)×1.0W=1.5 J
Answer: 10
(a)
a=F2 M+m (given)
The free-body diagrams of both the blocks are shown below:
For the block of mass m,
ma=μ1R1 and R1=mg⇒μ1=maR1=F2 M+m g
(b)
Frictional force acting on the smaller block,
f1=μ1R1=F2 M+m g×mg=mF2 (M+m)
(c) Work done, w = f1s [where s = d]
=mF2 M+m×d=mFd2 M+m
Answer: 11
Given:
Weight=2000 N, s=20 m, μ=0.2
The free-body diagram for the box is shown below:
(a) From the figure,
R+P sin θ-2000=0 … (i)P cosθ-0.2 R=0 .. (ii)
From (i) and (ii),
P cosθ-0.2 2000-P sin θ=0P cos θ+0.2 sinθ=400 P=400 cos θ+0.2 sin θ … (iii)
So, work done by the person,
W=PS cos θ=8000 cosθ cosθ+0.2 sinθ=80001+0.2 tanθ=40000 5+tanθ …(iv)
(b) For minimum magnitude of force from equation (iii),
ddk cos θ+0.2 sin θ=0⇒ tan θ=0.2
Putting the value in equation (iv),
W=400005+tan θ=400005+0.2≈7692 J
Answer: 12
Given:Weight, mg=100 Nθ=37° and s=2m Force, F=mg sin 37°= 100×35=60 N
So, work done when the force is parallel to incline,
W=FS cos θ=60×2×cos 0° =120 J
In ΔABC,AB=2 m AC=h=s×sin 37°=2.0×sin 37°=1.2 m
∴ Work done when the force is in horizontal direction,
W’=mgh=100×1.2=120 J
Answer: 13
Given:Mass of the car,m=500 kg Distance covered by the car,s=25 m Initial speed of the car,u=72 km/h=20 m/s Final speed of the car, ν=0 m/s
Retardation of the car,
a=ν2-u22 s⇒ a=-40050=-8 m/s Frictional force, F=ma=500×8=4000 N
Answer: 14
Given: Mass of the car, m=500 kg Initial velocity of the car, u=0 Final velocity of the car,ν=72 km/h=20 m/s a=ν2-u 22 s a=40050=8 m/s
Force needed to accelerate the car,
F=ma=500×8=400N
Answer: 15
Given,
ν=ax uniformly accelerated motion Displacement, s=d-0=d Putting x=0,
we get ν1=0Putting x=d, we get ν2=adα=ν22-ν122 s=a2d2d=a22
Force, F=mα=ma 22 Work done, W=Fs cos θ=ma 22×d=ma2d2
Answer: 16
(a)
Given:Mass of the block, m=2 kg θ=37° Force on the block, F=20 N
(b) If W=40 J
S=WF=4020=2 m h=2 sin 37°=1.2 m
So, work done
W=-mg h=-20×1.2=-24 J
(c)
ν=u+at=4×1=4 m/sec So,K.E.=12 mv 2=12×2×16=16 J
Answer: 17
Given:
Mass, m=2 kg Inclination, θ=37°Force applied, F=20 N Acceleration of the block, a=10 m/s
(a) t = 1 sec
So, s=ut+12 at 2=5 m
Work done by the applied force,
W=Fs cos θ°=20×5=100 J
(b) AB h=5 sin 37°=3 m
So, work done by weight,
W=mgh2×10×3=60 J
So, frictional force,
f=mg sin θ
Work done by the friction forces,
W=fs cos 0°=mg sin θ s=20×0.60×5=-60 J
Answer: 18
Given:Mass of the block, m=250 gm=0.250 kg Initial speed of the block, u=40 cm/s=0.4 m/s Final speed of the block, ν=0 Coefficient of friction, μ=0.1
Force in the forward direction is equal to the friction force.
Here, μR=ma where a is deceleration a=μRm=μmgm=μg=0.1×9.8=0.98 m/s s=ν2-u22 a=0.082 m= 8.2 cm
Again, work done against friction,
W=-μ Rs cos θ=-1×2.5×0.082×1=-0.02 J⇒ W=-0.02 J
Answer: 19
Given:Height,h=50m Mass of water falling per hour,m=1.8×105 kg
Power of a lamp,
P=100 watt Potential energy of the water,P.E.=mg h=1.8×105×9.8×50=882×105 J
As only half the potential energy of water is converted into electrical energy,
Electrical energy=12P.E.=441×105 J/hr
So, power in watt J/sec=441×10560×60
Therefore, the number of 100 W lamps that can be lit using this energy,
n=441×1053600×100=122.5≈122
Answer: 20
Given:Mass of the bucket with the paint, m=6 kg Height at which the bucket is placed, h=2 m potential energy of the bucket with the paint at the given height, P.E.=mgh =6×9.8×2=117.6 JP.E. on the floor=0 Loss in potential energy=117.6-0=117.6 J≈118 J
Answer: 21
Given:
Height of the cliff, h = 40 m
Initial speed of the projectile, u = 50 m/s
Let the projectile hit the ground with velocity ‘v‘.
Applying the law of conservation of energy,
mgh+12mu2=12mv2⇒10 × 40 + 12× 2500=12 v2⇒v2=3300⇒v=57.4
m/s=58 m/s
The projectile hits the ground with a speed of 58 m/s.
Answer: 22
Time taken to cover 200 m, t=1 min 57.56 seconds=117.56 s Power exerted by her, P=460 WP=Wt Work done, W=Pt=460×117.56 J Again, W=Fs⇒F=Ws=460×117.56200=270.3 N≈270 N
∴ Resistance force offered by the water during the swim is 270 N.
Answer: 23
Given:
Distance covered by her, s = 100 m
Time taken by her to cover 100 m, t = 10.54 s
Mass, m = 50 kg
The motion can be assumed to be uniform.
(a)
Speed, ν=st=9.487 m/sSo, K.E.=12mν2=2250 J
(b)Weight =mg =490 J Average resistance force offered,
R=mg 10=49 J So,work done against the resitance force W=-Rs=-49×100
⇒W=-4900 J
(c)
To maintain uniform speed, she had to exert 4900 J of energy to overcome friction.
Power exerted by her to overcome frcition,
P=Wt=490010.54=465 W
Answer: 24
Given:
Height through which water is lifted, h = 10 m
Flow rate of water=mt=30 kg/min=0.5 kg/s
Power delivered by the engine,
P=mght=0.5×9.8×10=49 W
1 hp = 746 w
So, the minimum horse power (hp) that the engine should possess
=p746=49746=6.6×10-2 hp
Answer: 25
Given, Mass of the stone, m=200 g=0.2kg Height to which the stone is lifted, h=150 cm= 1.5 m Velocity of the projection, ν=3 m/s Time, t=1 s Total work done, W=K.E.+P.E.W=12mν
2+mg h= 12×0.2 ×9 + 0 .2 9 .8×1.5=3.84 J
1 hp = 764 watt
Horsepower used by demonstrator
=3.84746=5.14×10-3
Therefore, power used by the demonstrator to lift and throw the stone is 5.14×10-3 hp.
Answer: 26
Given:
Mass of the metal, m=2000 kg
Distance, s = 12 m
Time taken, t = 1 minute = 60 s
Force applied by the engine to lift the metal,
F = mg
So, work done by the engine, W=F×s×cosθ= mgs×cos 0°[θ=0° for minimum force]= 2000×10×12=240000 J So, power exerted by the engine,P=Wt=24000060=4000 watt Power in hp,P= 4000746=5.3 hp
Answer: 27
The specifications given by the company are:
Mass, m=95 kg Maximum power,Pm=3.5 hp Maximum speed, vm=60 km/h=503 m/s Pick up time to get maximum speed, tm=5 sec
So, the maximum acceleration that can be produced,
a=503×5=103 m/s2
So, the driving force,
F=ma=95×103=9503 N Max speed, ν=pF
⇒v=3.5×746×3950 ⇒8.2 m/s
As the scooter can reach a maximum of 8.2 m/s while producing a force of 950/3 N, the specifications given are not correct.
Exercise : Solution of Questions on page Number : 134
Answer: 28
Given,Mass of the block, m=30 kg Speed acquired by the block,ν=40 cm/s=0.4 m/s Distance covered by the block, s=2 m
Let a be the acceleration of the block in the downward direction.
From the diagram, the force applied by the chain on the block,
F=ma-mg=m a-g
a=ν2-u22 s=16-4=0.04 m/s2 Work done by the chain,W=Fs cos θ
=m a-g×s cos 0° =30 0.04-9.8×2 =-30×9.76×2 =-585.6=-586 J⇒W=-586 J
Answer: 29
Given,Tension in the string
,T=16 N From the free-body diagrams,
T-2 mg+2 ma=0 … (i)
T-mg-ma=0 … (ii)
From equations (i) and (ii),
T=4 ma⇒a=T4m=4m m/s
Now, S=ut+12at2=12× 4m × 1
as u=0=2 m Net mass=2 m-m=m Decrease in
potential energy, P.E.=mgh=m×g×2m=9.8×2=19.6 J
Answer: 30
Given, m1=3 kg, m2=2 kg, t=during 4th second
From the free-body diagram,
T-3g+3a=0 … (i)T-2g-2a=0 … (ii)
Equating (i) and (ii), we get:
3g-3a=2g+2a⇒a=g5 m/s2
Distance travelled in the 4th second,
s(4th)=a2 2n-1=g522×4-1=7g10=7×9.810 m
Net mass ‘m’=m1-m2=3-2=1 kg
So, decrease in potential energy,
P.E. = mgh
P.E.=1×9.8×710×9.8=67.2 J=67 J
So, work done by gravity during the fourth second = P.E.= 67 J
2Answer: 31
Given,m1=4 kg, m2=1 kg,v2=0.3 m/s v1=2×0.3=0.6 m/s v1=2v2 in this system Height descended by the 1 kg block,h=1 m Distance travelled by the 4 kg block,s=2×1=2 m Initially the system is at rest. So, u=0 Applying work energy theorem which says that change in K.E.= Work done for the system 12 m1ν12+12 m2ν22=-μR s+m2gh
12×4×0.36+12×1×0.09 [As, R=4g=40 N]=-μ 40×2+1×40×1⇒ 0.72+0.045=-80 μ+10⇒μ=9.23580=0.12 So, the coefficient of kinetic friction between the block and the table is 0.12 .
Answer: 32
Given,Mass of the block, m=100 g=0.1 kg,Velocity of the block at the highest point, ν=5 m/s Radius of the circular tube, r=10 cm
Work done by the block = Total energy at the highest point − Total energy at the lowest point
=12mν2+mgh-0
⇒W=12×0.1×25+0.1×10×0.2As,
h=2r=0.2 mW=1.25+0.2=1.45 J
So, the work done by the tube on the body is 1.45 joule.
Answer: 33
Given,Mass of the car, m=1400 kgh=10 m Since the car is moving when the motor stops, it has kinetic energy. Thus vi=54 km/h×518=15 m/svf=0△K=12mvf2-12mvi2△K=12×140002-152△K=-157500 J.Let the gravitational potential energy be zero at the starting point.Then the potential energy at the terminal is Ui=0Uf=mghUf=1400×9.8×10=137200 J△U=Uf-Ui△U=137200-0=137200 J Let W be the work done against friction during ascent. Then ” W” is the work done by the frictional force.
-W=△K+△U-W=-157500+137200-W=-20300J W=20300 J
So, work done against friction is 20,300 joule.
Answer: 34
Given, Mass of the block, m=200 g=0.2 kg Length of the incline, s=10 m, Height of the incline, h=3.2 m Acceleration due to gravity, g=10 m/s
(a)
Work done, W = mgh = 0.2 × 10 × 3.2 =6 .4 J
(b)
Work done to slide the block up the incline
W=mg sin θ×s=0.2×10×3.2/10×10=6.4 J
(c)
Let final velocity be v when the block falls to the ground vertically.
Change in the kinetic energy = Work done
12mv2-0=6.4 J
⇒ ν=8 m/s
(d)
Let ν be the final velocity of the block when it reaches the ground by sliding.
12mν2-0=6.4 J
⇒ v=8 m/s
Answer: 35
Given,Length of the slide, l=10m Height of the slide, h=8 m Weight of the boy, mg=200 N
Friction force,
F=200×310=60 N
(a) Work done by the ladder on the boy is zero, as work is done by the boy himself while going up.
(b) Work done against frictional force,
W=μ RS=fl= -60×10 =-600 J
Answer: 36
Given,
Height of the starting point of the track, H = 1 m
Height of the ending point of the track, h = 0.5 m
Let v be the velocity of the particle at the end point on the track.
Applying the law of conservation of energy at the starting and ending point of the track,we get
mgH=12mν2+mgh⇒g-12ν2=0.5 g⇒ν2=2 g-0.5 g=g⇒ ν=g=3.1 m/s
After leaving the track, the body exhibits projectile motion for which, θ=0y=-0.5 Using equation of motion along the horizontal direction, -0.5 = u sin θ t – 12 gt2⇒0.5=4.9×t2⇒t=0.31 sec So, x=ν cosθ t =3.1 × 0.31=1 m
So, the particle will hit the ground at a horizontal distance of 1 m from the other end of the track.
Answer: 37
Given, Weight of the block, mg=10 N Friction coefficient, μ=0.2 Initial height of the block,H=1 m Initial velocity = Final velocity=0
Potential energy of the block at the top of the curved track = Kinetic energy of the block at the bottom of the track
⇒ K.E.=mgh=10×1=10 J
Again on the horizontal surface the frictional force,
F=μR=μmg=10×1=10 J
So, the K.E. is used to overcome friction.
⇒S=WF=10 2 =5 m
The block stops after covering 5 m on the rough surface.
Answer: 38
Let ‘dx‘ be the length of an element at distance x from the table.
Mass of the element, ‘dm‘=ml dx
Work done to putting back this mass element on the table is
dW=ml×x×g×dx
So, total work done to put 13 part back on the table
W=∫01/3ml gx dx
⇒W=ml gx221/3=mgl18 l=mgl18
The work to be done by a person to put the hanging part back on the table is mgl18.
Answer: 39
Let x length of the chain be on the table at a particular instant.
Consider a small element of length ‘dx’ and mass ‘dm‘ on the table.
dm = MLdx
Work done by the friction on this element is
dW=μ Rx=μ ML×gxdx
Total work done by friction on two third part of the chain,
W=∫2L/30 μML gx dx
∴ W=μMLg x2202L/3=-μMLg 4L218=-2μMgL9
The total work done by friction during the period the chain slips off the table is -2μMgL9.
Answer: 40
Given, Mass of the block, m=1 kg Height of point A, H=1 m Height of point B, h=0.8 m
Work done by friction = Change in potential energy of the body
⇒ W1=mgh-mgH=1×10 0.8-1=-1×10×0.2=-2 J
The work done by the frictional force on the block during its transit from A to B is -2 joule.
Exercise : Solution of Questions on page Number : 135
Answer: 41
Given, Mass of the block, m=5 kg Compression in the string with the load, x=10 cm=0.1 m Initial speed in upward direction, ν=2 m/s, h=?, g=10 m/sec So, F=kx=mg⇒k=mgx⇒500.1=500 N/m
Total energy just after the impulse,
E=12mν2+12kx2 … (i)
Total energy at a height h
=12k h-x2-mgh
On solving, we get:
h = 0.2 m
= 20 cm
Answer: 42
Given, Mass of the block, m=250 g=0.25 kg, Spring constant, k=100 N/m Compression in the string, x=10 cm=0.1 m, Acceleration due to gravity, g=10 m/s
Let the block rises to height h.
Applying law of conservation of energy which says that the total energy should always remain conserved.
12kx2=mgh⇒
h=12kx2mg=100×0.012× 0.250×10=0.2 m=20 cm
So, the block rises to 20 cm.
Answer: 43
Given:Mass of the block, m=2 kg Initial distance of the block from the spring, S1=4.8 m, Comression in the spring, x=20 cm=0.2 m Final distance of the block from the spring, S2=1 mAs θ=37°,sin 37°=0.60=35cos 37°=0.80=45
Applying the work-energy principle for downward motion of the block,
0-0=mg sin 37° x+4.8-μR×5-12kx2⇒20×0.06×5-μ×20×0.80×5-12k 0.22=0⇒60-80 μ-0.02 k=0⇒80 μ+0.02 k=60 … (i)
Similarly for the upward motion of the body the equation is
0-0=-mg sin 37°-μR×1+12k -22⇒20×0.06×1-μ×20×0.80×1-12k 0.22⇒12-16 μ+0.02 k=0
Adding equations (i) and (ii), we get:
96 μ=48⇒ μ=0.5
Now putting the value of μ in equation (i), we get:
k = 1000 N/m
Answer: 44
Let the velocity of the body at P be ν.
So, the velocity of the body at Q is ν2.
Energy at point P = Energy at point Q
So, 12 mν P2-12 mvQ2=12kx2
⇒12kx2=12m VP2-VQ2⇒kx2= m ν2-ν24⇒kx2=m 4ν2-ν24⇒k=3mv24x2
Answer: 45
Mass of the body is m.
Let the elongation in the spring be x.
Applying the law of conservation of energy,
12kx2=mgx⇒x=2 mg/k
Answer: 46
The body is displaced x towards the right.
Let v be the velocity of the body at its mean position.
Applying the law of conservation of energy,
12mν2=12k1x2+12k2x2
⇒mν2=x2k1+k2⇒ν2=x2 k1+k2m⇒ν=xk1+k2m
Answer: 47
Let the compression in the spring be x.
(a) Applying the law of conservation of energy,
maximum compression in the spring will be produced when the block comes to rest .
so change in kinetic energy of the block due to change in its velocity from u m/s to 0 will be equal to the gain in potential energy of the spring.
change in kinetic energy of the block=12mv2-12m(0)2=12mv2
gain in the potential energy of spring=12kx2
12mν2=12kx2⇒ x2=mν2kx=νmk
(b) No. The velocity of the block will not be same when it comes back to the original position. It will be in the opposite direction and the magnitude will be the same if we neglect all losses due friction and spring to be perfectly elastic.
Answer: 48
Given: Mass of the block, m=100 g=0.1 kg Compression in the spring, x=5 cm=0.05 m Spring constant, k=100 N/m
Let v be the velocity of the block when it leaves the spring.
Applying the law of conservation of energy,
Elastic potential energy of the spring = Kinetic energy of the block
12mν2=12kx2⇒ ν=xkm=0.05×1000.1=1.58 m/s
For the projectile motion,
θ=0°, y=-2Now, y= u·sin θ t-12gt2-2=-12×9.8×t2
⇒ t=0.63 sec,So, x=u cos θ t=1.58×0.36=1 m
Therefore, the block hits the ground at 1 m from the free end of the spring in the horizontal direction.
Answer: 49
Let the velocity of the body at L is ‘ν’ .
If the body is moving in a vertical plane then we need to find the minimum horizontal velocity which needs to be given to the body (velocity at L).
Also as point H is the highest point in the vertical plane so horizontal velocity at H will be zero.
Applying law of conservation of energy at points L and H,
12mν2=mgh12mν2=mg 2L⇒ν=4 gL=2 gL
Answer: 50
Given:Mass of each block, m=320 g=0.32 kg Spring constant, k=40 N/m h=40 cm=0.4 m and g=10 m/s
From the free-body diagram,
kx cos θ=mg
As, when the block breaks of the surface below it (i.e. gets dettached from the surface) then R =0.
⇒ cosθ=mgkx⇒0.40.4+x=3.240 x
⇒ 16x=3.2 x+1.28
⇒x=0.1 m So,s=AB=h+x2-h2=0.52-0.42=0.3 m
Let the velocity of body B be ν.
Change in K.E. = Work done (for the system)
12 mu2+12mν2=-12kx2+mgs
⇒0.32×ν2=-12×40×1.02+0.32×10×0.3
⇒ν=1.5 m/s
From the figure,
∆l=h sec θ-1 … i
From the principle of conservation of energy,mgs=212mν2+12K ∆l2mgh
tan θ=mν2+12kh2 sec θ-12 … (ii)
When the motion of the block breaks of the surface below it (i.e gets dettached from the surface on which it was initially placed) then
mg=kh sec θ-1 cos θ⇒1-cos θ=mgkh⇒ cos θ=1-mgkhor cos θ=kh-mgkh=40×0.4-0.32×1040×0.4=0.8
Putting the value of θ in equation (ii), we get:
0.32×10×0.4×0.75
=0.32 ν2+1240×0.42 1.25-12⇒0.96=0.32 ν2+0.2⇒0.32 ν2=0.72⇒ν=1.5 m/s
Exercise : Solution of Questions on page Number : 136
Answer: 51
θ=37°, l= natural length h
Let the velocity be ‘ν’.
cos 37°=BCAC=0.8=45AC=h+x=5h4
Applying the law of conservation of energy,
12kx2=12mν2⇒ν=xkm=h4 km
Answer: 52
Let v be the minimum velocity required to complete a circle about the ring.
Applying the law of conservation of energy,
Total energy at point A = Total energy at point B
mgl+12mv2=mg(2l)+0⇒v=2gl
Let the rod be released from a height h.
Total energy at A = Total energy at B
mgh=12mν2mgh=12m 2 gl
So, h = l
Answer: 53
(a) Let the velocity at B be v1.12mν2=12mv12+mgl
⇒12m 10 gl=12mν12+mglν12=8 gl
So, the tension in the string at the horizontal position,
T=mν2R=m8 gll= 8 mg
(b) Let the velocity at C be v2.
12mν2=12mν22+mg (2l)
⇒ 12m 10 gl=12mν22+2mgl⇒ν22=6 gl
So, the tension in the string is given by
TC=mv22l-mg=5 mg
(c) Let the velocity at point D be ν4.Again, 12mν2=12mν32+mgl 1+cos 60°
⇒ν32=7 gl
So, the tension in the string,
TD=mν32l-mg cos 60°=m7 gll-0.5 mg= 7 mg-0.5 mg=6.5 mg
Answer: 54
From the figure,
cos θ=OCOB⇒OC=OB cos θ=0.5×0.8=0.4 So, CA=0.5-0.4=0.1 m
Total energy at A = Total energy at B
12 mν2=mg ACν2=2×10×0.1=2⇒v=2
So, the tension is given by
T=mν2r+mg=0.1 20.5+10=1.4 N
Answer: 55
Given,
normal force on the track at point P,
N = mg
As shown in the figure,
mν2R=mg⇒ν2=gR … (i)
Total energy at point A = Total energy at point P
i.e. 12kx2=12mν2+mgR⇒x2=mgR+2mgRk [because, ν2=gR]⇒x2=3 mgR/k⇒x=3mgRk
Answer: 56
Suppose the string becomes slack at point P.
Let the bob rise to a height h.
h = l + l cos θ
From the work-energy theorem,
12 mν2-12mu2=-mghν2=u2-2g l+l cos θ … (i)
Again, mν2l=mg cos θν2=lg cos θ ………..(ii)
Using equation (i) and (ii) and the value of u, we get,
gl cos θ=3gl-2gl-2gl cos θ3 cos θ=1θ=cos-1 13=cos-1 -13
Answer: 57
Given: Length of the string, L=1.5 m Initial speed of the particle, u=57 m/s(a) mg cosθ=mν2 Lν2=Lg cosθ …(i)
Change in K.E. = Work done
12mν2-12mu2=-mgh⇒ν2-57=-2×1.5 g 1+cos θ ⇒ ν2=57-3g 1+cos θ …(ii)
Putting the value of ν from equation (i),
15 cos θ=57-3g 1+cos θ⇒ 15 cos θ=57-30-30 cos θ⇒ 45 θ=27⇒ cos θ=35⇒ θ=cos-1 35=53°
(b) From equation (ii),
ν=57-3g 1+cos θ =9=3 m/s
(c) As the string becomes slack at point P, the particle will start executing a projectile motion.
h=OF+FC=1.5 cosθ+u2 sin2θ2
g=1.5×35+9×0.822×10=1.2 m
Answer: 58
Applying the law of conservation of emergy
12 mνc2-0=mg L2 1-cos α
[because, distance between A and C in the vertical direction is L2 and
L2=1-cos α⇒ Vc2=gL 1-cos α… (i)
Again, from the free-body diagram (fig. (ii)),
mνc2L/2=mg cos α .. (ii)
[because, Tc = 0]
From equations (i) and (ii),
gL 1-cos α=gL2 cos α⇒ 1-cos α=12 cos α⇒ 32 cos α=1⇒ cos α=23 … (iii)
To find highest position C1 upto which the bob can go before the string becomes slack.(as we have found out the value of α so now we want to find the distance of the highest point upto which the bob goes before the string becomes slack,using this value of α.
BF=L2+L2 cos θ=L2+L2×23=L 12+13So, BF=5L6
(c) If the particle has to complete a vertical circle at the point C,
mνc2L-x=mg … (i)
Again, applying energy conservation principle between A and C
12 mνc2-0=mg OC⇒12 mνc2=mg L-2 L-x=mg 2x-L⇒ νc2=2g 2x-L … (ii)
From equations (i) and (ii),
gL-x=2g 2x-L⇒L-x=4x-2L⇒5x=3L
∴ xL=35=0.6So, the minimum value of xL shoule be 0.6.
Answer: 59
Let the velocity be ν when the body leaves the surface.
From the free-body diagram,
mν2R=mg cos θ [normal reaction]ν2=Rg cos θ … (i)
Again, from the work-energy principle,
Change in K.E. = Work done
⇒12mν2-0=mg R-R cos θ⇒ν2=2gR 1-cos θ … (ii)
From (i) and (ii),
Rg cos θ=2gR 1-cos θ
3gR cos θ=2gRcos θ=23θ=cos-1 23
Answer: 60
(a) When the particle is released from rest, the centrifugal force is zero.
So, mν2R=mg cos θ⇒ ν2=Rg cos θ .. (i)
Again, 12 mν2=mg R cos 30°-cos θ⇒ν2=2Rg 32-cos θ … (ii)
From equations (i) and (ii),
Rg cos θ=2 Rg 32-cos θ
⇒ 3 cos θ=3⇒ cos θ=13or θ=cos-1 13
So, the distance travelled by the particle before losing contact,
L=R θ-π6 because 30°=π6
Putting the value of θ, we get:
L = 0.43 R
Answer: 61
(a) Radius = R
Horizontal speed = ν
From the above diagram:
Normal force,
N=mg-mv2R
(b) When the particle is given maximum velocity, so that the centrifugal force balances the weight, the particle does not slip on the sphere.
So,mν2R=mg⇒ν=gR
(c) If the body is given velocity ν1 at the top such that,
ν1=gR2ν12=gR4
Let the velocity be ν2 when it loses contact with the surface, as shown below.
So, mν22R=mg cos θ⇒ν22=Rg cos θ … (i)
Again, 12 mν22-12 mν12=mgR 1-cos θ⇒ν22=ν12+2gR 1-cos θ … (ii)
From equations (i) and (ii),
Rg cos θ=Rg4+2gR 1-cos θ⇒ cos θ=14+2-2 cos θ⇒ 3 cos θ=94⇒ θ=cos-1 34
Exercise : Solution of Questions on page Number : 137
Answer: 62
(a) Net force on the particle at A and B,
F=mg sin θ
Work done to reach B from A,
W=FS=mg sin θl
Again, work done to reach B to C
=mgh=mg R 1-cos θ
So, total work done
=mgl sin θ+mgR 1-cos θ=mgl sin θ+R 1-cos θ
Let the velocity at C be ν0.
Applying energy principle,
12 mν02-12 m2ν02=-mg l sin θ+R 1-cos θ⇒ V2=4ν02-2g l sing θ+R 1-cos θ=4.2 g l sin θ+R 1-cos θ-2g l sin θ+R 1-cos θ
So, force acting on the body,
N=V2R=6 mg lR sin θ + 1-cos θ
(c) Let the loose contact after making an angle θ.
mν2R=mg cos θ⇒ ν2=Rg cos θ … (i)
Again, 12mν2=mg R-R cos θ⇒ν2=2gR 1-cos θ … (ii)
From (i) and (ii),=cos-1 23⇒θ=cos-1 23
Answer: 63
Let us consider a small element, which makes angle ‘dθ’ at the centre.
∴ dm=ρ mL Rdθ
(a) Gravitational potential energy of ‘dm‘ with respect to centre of the sphere
=dm g R cos θ= mgL R2 cos θ dθ
∴ Total gravitational potential energy, EP=∫0L/RmgR2L cosθ dθ EP=mR2gL sinθ As, θ=LR EP=mR2gL sin LR
(b) When the chain is released from rest and slides down through an angle θ,
Change in K.E. of the chain = Change in potential energy of the chain
=mR2gLsin LR-∫gR2L cos θ dθ=mR2gLsin LR+sin θ-sin θ+LR
(c) Since,
K.E.=12mν2=mR2gL sin LR
Taking derivative of both sides with respect to ‘t‘, we get:
12×2ν×dνdt=R2gLcos θ-dθdt-cos θ+LRdθdt
∴ R-dθdtdvdt=R2gL×dθdtcos θ-cos θ+LR because ν=Rω=Rdθdt
∴ dνdt=RgLcos θ-cos θ+LR
When the chain starts sliding down,
θ=0°
∴dνdt=RgL1-cos LR
Answer: 64
Suppose the sphere moves to the left with acceleration ‘a‘
Let m be the mass of the particle.
The particle ‘m‘ will also experience inertia due to acceleration ‘a‘ as it is in the sphere. It will also experience the tangential inertia force mdνdt and centrifugal force mν2R.
From the diagram,
mdνdt=ma cos θ+mg sin θ
⇒ mνdνdt=ma·cos θ Rdθdt+mg sin θ Rdθdt because, ν=Rdθdt⇒ν dν=a R cos θ dθ+gR sin θ dθ
Integrating both sides, we get:
ν22=aR sin θ-gR cos θ+C
Given : θ=0, ν=0
So, C=gR
⇒ν22=aR sin θ-gR cos θ+gR⇒ν2=2R a sin θ + g-g cos θ⇒ ν=2R a sin θ+g-g cos θ1/2