Free NCERT Solutions for Class 12 Maths Chapter 2 Inverse Trigonometric Functions solved by Expert Teachers as per NCERT (CBSE) Book guidelines and brought to you by Toppers Bulletin. These Inverse Trigonometric Functions Exercise Questions with Solutions for Class 12 Maths covers all questions of Chapter Inverse Trigonometric Functions Class 12 and help you to revise complete Syllabus and Score More marks as per CBSE Board guidelines from the latest NCERT book for class 12 maths. You can read and download NCERT Book Solution to get a better understanding of all topics and concepts.
2.1 Introduction
2.2 Basic Concepts
2.3 Properties of Inverse Trigonometric Functions.
Inverse Trigonometric Functions NCERT Solutions – Class 12 Maths
Q1 : Find the principal value of 
Answer :
Let =y. Then sin y=
We know that the range of the principal value branch of sin-1 is
and sin
Therefore, the principal value of
Q2 : Find the principal value of
Answer :
We know that the range of the principal value branch of cos -1is
Therefore, the principal value of
.
Q3 : Find the principal value of cosec-1(2)
Answer :
Let cosec -1(2) = y. Then,
We know that the range of the principal value branch of cosec-1is 
Therefore, the principal value of
Q4 : Find the principal value of
Answer :
We know that the range of the principal value branch of tan -1is
Therefore, the principal value of
Q5 : Find the principal value of
Answer :
We know that the range of the principal value branch of cos -1is
Therefore, the principal value of
Q6 : Find the principal value of tan-1(-1)
Answer :
Let tan-1(-1) = y. Then,
We know that the range of the principal value branch of tan-1 is
Therefore, the principal value of
Q7 : Find the principal value of
Answer :
We know that the range of the principal value branch of sec-1 is
Therefore, the principal value of
Q8 :Find the principal value of
Answer :
We know that the range of the principal value branch of cot-1 is
(0,π) and 
Therefore, the principal value of
Q9 : Find the principal value of
Answer :
We know that the range of the principal value branch of cos-1 is [0,π] and
Therefore, the principal value of
Q10 : Find the principal value of
Answer :
We know that the range of the principal value branch of cosec-1 is
Therefore, the principal value of
Q11 :Find the value of
Answer :
Q12 :Find the value of
Answer :
Q13 :Find the value of if sin – 1 x = y, then
(A)
(B)
(C)
(D)
Answer :
It is given that sin-1 x = y.
We know that the range of the principal value branch of sin-1 is
Therefore,
.
Q14 :Find the value of
is equal to
(A) π (B) 
(C)
(D)
Answer
Exercise 2.2 : Solutions of Questions on Page Number : 47
Q1 :Prove 
Answer :
To prove:
Let x = sinθ. Then, 
We have,
R.H.S. =
= 3θ
= L.H.S.
Q2 :Prove
Answer :
To prove:
Let x = cosθ. Then, cos-1 x =θ.
We have,
Q3 :Prove 
Answer :
To prove:
Q4 :Prove 
Answer :
To prove:
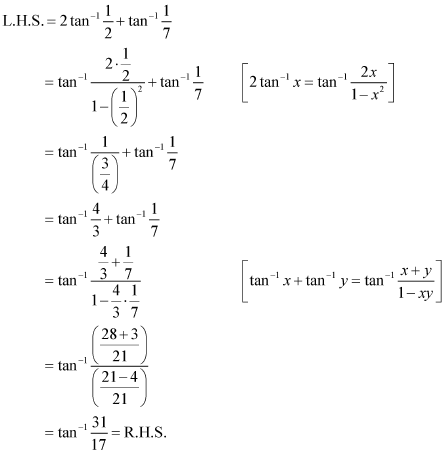
Q6 :Write the function in the simplest form:
Answer :
Put x = cosec θ ⇒ θ = cosec-1 x
Q7 :Write the function in the simplest form:
Answer :
Q8 :Write the function in the simplest form:
Answer :
Q9 :Write the function in the simplest form:
Answer :
Q10 :Write the function in the simplest form:
Answer :
Q11 :Find the value of
Answer :
Let
. Then,
Q12 :Find the value of 
Answer :
Q13 :Find the value of
Answer :
Let x = tan θ. Then, θ = tan-1 x.
Let y = tan Φ. Then, Φ = tan-1 y.
Q14 :If,
then find the value of x.
Answer :
On squaring both sides, we get:
Hence, the value of x is
Q15 :If, 
then find the value of x.
Answer :
Hence, the value of x is
Q16 :Find the values of 
Answer :
We know that sin-1 (sin x) = x if
, which is the principal value branch of sin-1 x.
Here,
Now,
can be written as:
Q17 :Find the values of
Answer :
We know that tan-1 (tan x) = x if,
which is the principal value branch of tan-1x.
Here,
Now,
can be written as:
Q18 :Find the values of
Answer :
Let. 
Then,
Q19 :Find the values of
is equal to
(A) 
(B)
(C)
(D)
Answer :
We know that cos-1 (cos x) = x if
, which is the principal value branch of cos-1 x.
Here,
Now,
can be written as:
The correct answer is B.
Q20 :Find the values of 
is equal to
Answer :
Let 
. Then 
We know that the range of the principle value branch of 
The correct answer is D.
Q21 :Find the values of
is equal to
(A) π (B) 
(C) 0 (D)
Answer :
Let. 
Then,
We know that the range of the principal value branch of
Let.
The range of the principal value branch of
The correct answer is B.
Exercise Miscellaneous : Solutions of Questions on Page Number : 51
Q1 :Find the value of
Answer :
We know that cos-1 (cos x) = x if
, which is the principal value branch of cos-1 x.
Here,
Now, 
can be written as:
Q2 :Find the value of
Answer :
We know that tan-1 (tan x) = x if
, which is the principal value branch of tan-1 x.
Here,
Now,
can be written as:
Q3 :Prove
Answer :
Now, we have:
Q4 :Prove
Answer :
Now, we have:
Q5 :Prove
Answer :
Now, we will prove that:
Q6 :Prove
Answer :
Now, we have:
Q7 :Prove
Answer :
Using (1) and (2), we have
Q8 :Prove
Answer :
Q9 :Prove
Answer :
Q10 :Prove
Answer :
Q11 :Prove 
[Hint: putx = cos 2θ]
Answer :
Q12 :Prove
Answer :
Q13 :Solve
Answer :
Q14: Solve 
Answer:
Q15 :Solve
is equal to
(A) 
(B)
(C)
(D)
Answer :
Let tan – 1 x = y. Then,
The correct answer is D.
Q16 :Solve,
then x is equal to
(A) 
(B) 
(C) 0 (D)
Answer :
Therefore, from equation (1), we have
Put x = sin y. Then, we have:
But, when
, it can be observed that:
is not the solution of the given equation.
Thus, x = 0.
Hence, the correct answer is C.
Q17 :Solve
is equal to
(A) 
(B) 
(C)
(D)
Answer :
Hence, the correct answer is C.